In a cirlce H, if angleDHG=(11x-36)^\circ and angleGHF=(x+12)^\circ, find hat{DG}? I've found x=17 and the angleDHG=151^\circ. Would you please help me on the next step to find the arc length?

1 Answer
Jan 22, 2018
You've already figured it out.
Explanation:
An arc’s length means the same commonsense thing length always means — you know, like the length of a piece of string (with an arc, of course, it’d be a curved piece of string).
Make sure you don’t mix up arc length with the measure of an arc which is the degree size of its central angle.
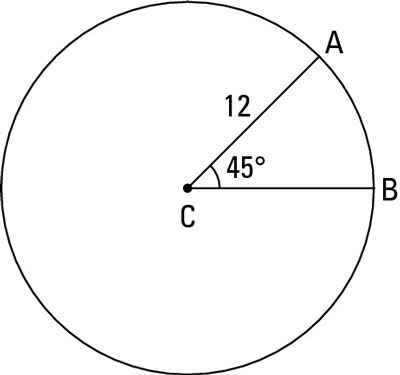
In this above given figure, we have measure of the arc
Remember, the degree measure of an arc is written like
If we were to find the length of the arc

