Question

Question #f36b9

Answer 1

Refer explanation.

Explanation:

Given -

`y=x^4+8x^3`

To graph, a function, first find the turning points.
Find the first derivative and set it to zero, so that you know the value of `x`, the slope of the curve becomes zero.

`dy/dx=4x^3+24x^2`

`dy/dx=0=>4x^3+24x^2=0`

`4x^2(x+6)=0`

`4x^2=0`

`x=0`

`x=-6`

At `x=0` and at `x=-6`, there are turning points.
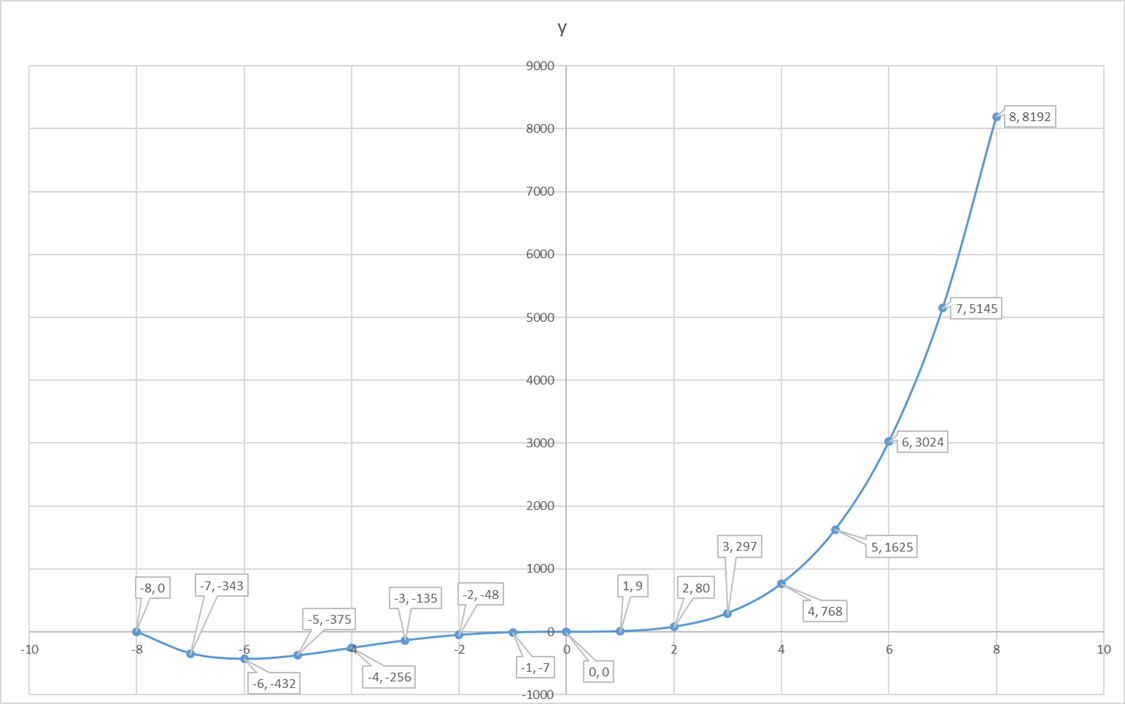
Take a range of values that includes 0 and -6. Calculate the corresponding value of `y`. Tabulate them. Use the pair of the points to obtain the curve.

At `x=0`, there is a point of inflexion.
At `x=-8`, the curve reaches the minimum.