How do you use the binomial series to expand #(2x^3 – 3)^10#?
1 Answer
Jan 29, 2018
As explained below
Explanation:
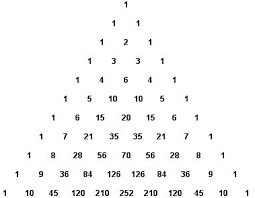
10th row of Pascal's triangle
1 10 45 120 210 252 210 120 45 10 1
As explained below

10th row of Pascal's triangle
1 10 45 120 210 252 210 120 45 10 1