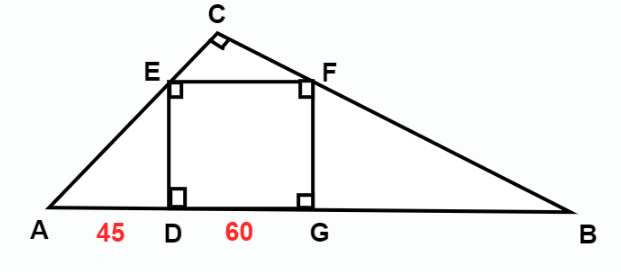
Given that, \overline{AD}=45 and \overline{DG}=60, find the lengths of other line segments in this right triangle \triangle ABC given that the DEFG is a square?
2 Answers
Explanation:

In triangle CEF,
In triangle BFG,
In triangle AEF,
Verification :
In right triangle ABC,
Hence Proved.
Please see below.
Explanation:
.
Because
Because
This means that by virtue of Angle Angle theorem:
Therefore, all four right angle triangles are similar which mean the ratios of their corresponding sides are equal.

