Question

Find the area of an equilateral triangle with apothem 7 cm? Round to the nearest whole number.

help me.

Answer 1

I got `28.07 \ "cm"^2`.

Explanation:

The apothem of the triangle will also be the triangle's height.

Let's work out the height of an equilateral triangle in terms of its sides.

Say we have an equilateral triangle of side `a`.

Its height will be the perpendicular bisector of another side, let's call it `b`. But since this triangle is equilateral, all of its sides are equal to one another, i.e. `a=b`.

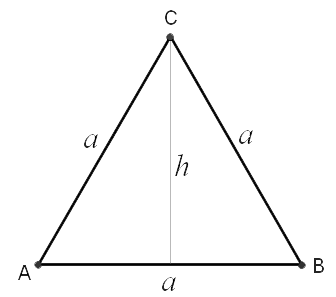
As you can see here, we split the triangle into `2` equal right triangles.

Now, let's work out the height `h` in terms of `a`.

We can use Pythagoras's theorem to work out the height, `h`.

We got:

`(a/2)^2+h^2=a^2`

`a^2/4+h^2=a^2`

`h^2=(3a^2)/4`

`h=sqrt((3a^2)/4)`

`h=(asqrt(3))/2`

So, the height of an equilateral triangle with sides `a` will be `(asqrt(3))/2`.

Now, we can put this formula back into our original problem.

We have the apothem or the triangle's height as `7 \ "cm"`. Since this triangle is equilateral, the one of its sides will have a length of

`(asqrt(3))/2=7`

`asqrt(3)=14`

`a=14/sqrt(3)`

`a~~8.08`

So, the triangle's side will be `8.08 \ "cm"` long.

The area of a triangle is given by `A=1/2a*h` (in this case).

Plugging in our values, we get

`A=1/2*8.08*7=28.07 \ "cm"^2`