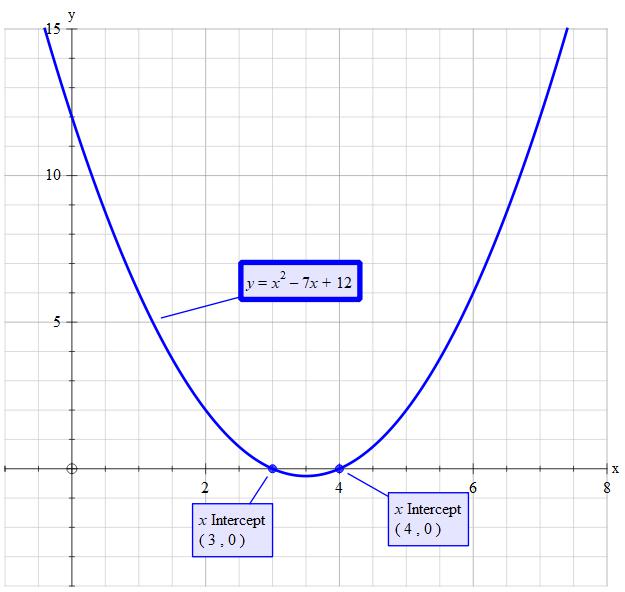
How do you factor this and use the zero product property to find the roots?
Factor and use the zero product to find the roots of the following quadratic equations.
a.) #0=x^2-7x+12#
b.) #0=6x^2-23x+20#
c.) #0=x^2-9#
d.) #0=x^2+12x+36#
Factor and use the zero product to find the roots of the following quadratic equations.
a.)
b.)
c.)
d.)
1 Answer
Feb 28, 2018
Only answering for question a
Explanation:
Given:
Lets look at the factors of 12
Need factors of 12 that we can sum to give
This one works
So we have:
Condition 1:
Condition 2: