Question

How do you find `\lim _ { x \rightarrow \infty } ( \frac { 5x + 9} { 7x ^ { 2} - 2x + 1} )`?

Answer 1

`0`

Explanation:

if the denominator is a higher degree polynomial than the numerator (`2>1` for this problem), then the limit as `xrarroo` will always be `0`

you can also check the value of `(5x+9)/(7x^2-2x+1)` at very large x-values and you will see a pattern:

`x=100rarr(5x+9)/(7x^2-2x+1)=.007...`
`x=1000rarr(5x+9)/(7x^2-2x+1)=.0007...`
`x=10000rarr(5x+9)/(7x^2-2x+1)=.00007...`

as x approaches `oo`, `(5x+9)/(7x^2-2x+1)` approaches `0`

you can also confirm with the graph: graph{(5x+9)/(7x^2-2x+1) [-10.15, 18.32, -6.84, 7.4]}

Answer 2

0

Explanation:

You can think about this in a couple of ways.

First, look at the polynomials in the numerator and denominator: which one is increasing faster?

The one in the denominator is, right? It's a 2nd degree polynomial, while the one in the numerator is 1st degree.

Hence, as `x` approaches infinity, the denominator will increase at a much faster rate than the numerator, which means that you'll be dividing by an increasingly larger and larger number, which means that your limit will go to 0.

---

If you prefer seeing this more algebraically, then we can use the method of dividing every term by the highest power in the expression. In this case, we have `x^2` as our highest power, so let's divide every term by that:

`lim_(x->oo) (5/x+9/x^2)/(7-2/x+1/x^2)`

You can now take the limit of each term in this expression.

Now, notice that you have a lot of terms where a constant is being divided by `x` or `x^2`. Again, think about what's happening as `x->oo`: you have some constant value that's being divided by an increasingly larger and larger quantity. This means that wherever we have this, the limit will be 0. So, let's put this in:

`=> (0+0)/(7-0+0)`

`= 0`

Hope that helped :)

Answer 3

Approach for exam use ....

:-)

Explanation:

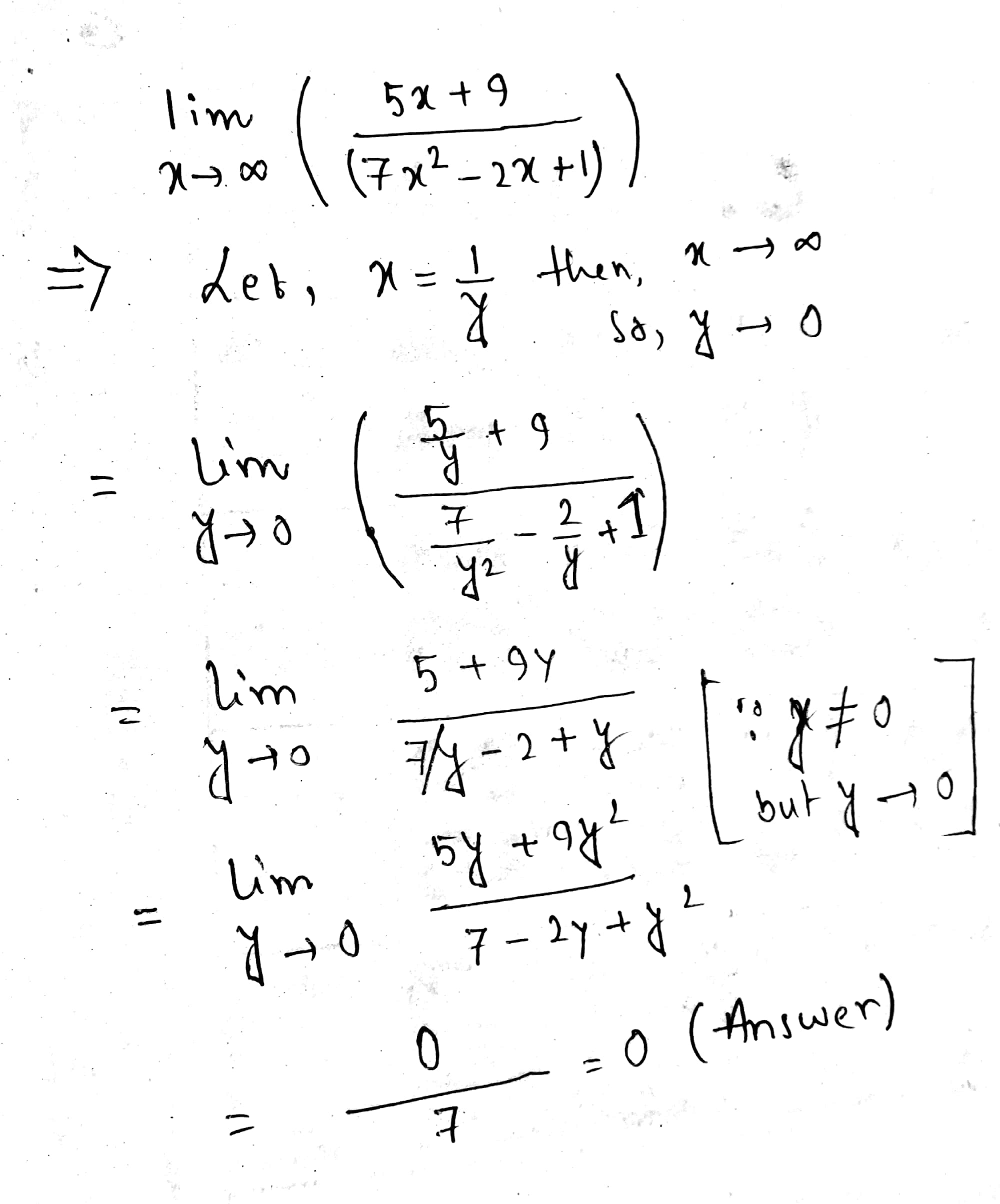
Direct approach... No language. For language , further answer referred.

Hope it helps...
Thank you...