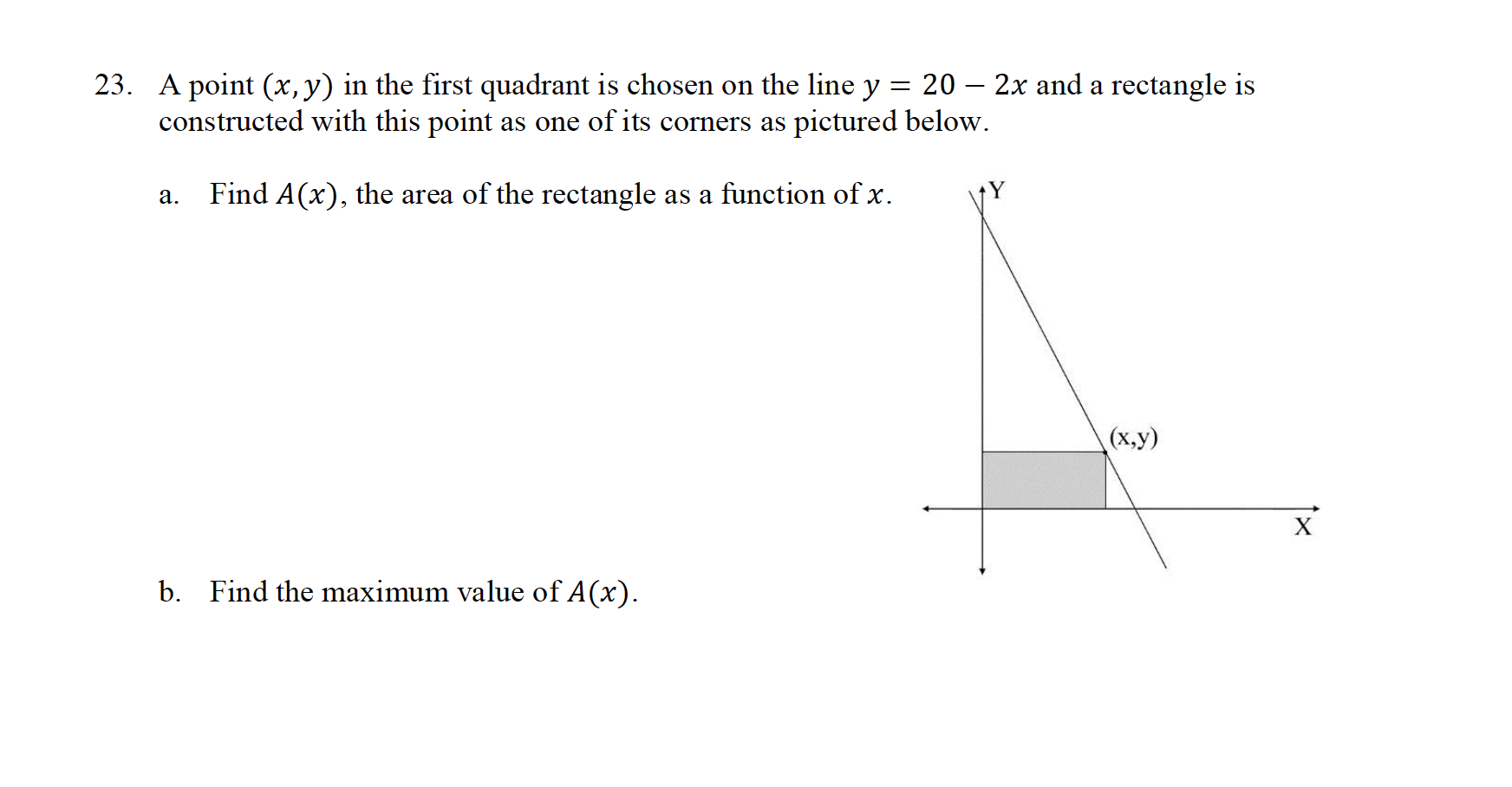
Finding A(x), the area of a rectangle as a function of x, and the maximum value of A(x)?
1 Answer
Apr 23, 2018
See explanation.
Explanation:
The sides of the rectangle are
#A=x*y=x*(20-2x)#
#A=-2x^2+20x#
graph{-2x^2+20x [-118.6, 118.6, -59.4, 59.3]}
From the above graph we see that the function
#p=(-b)/(2a)=(-20)/-4=5#
The maximum area is:
#A_max=5*(20-10)=50#
Answer:
a) The area is:
b) The maximum area is:
