Question

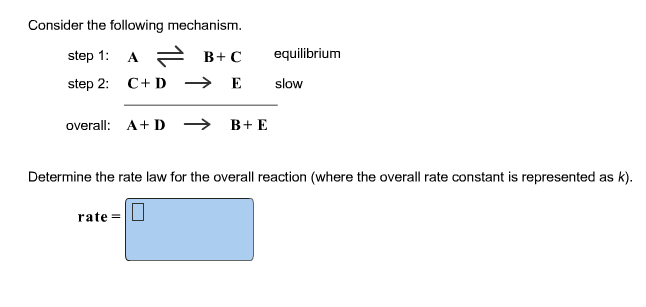
Determine the rate law for the overall reaction (where the overall rate constant is represented as k)?

Answer 1

I would get that `r(t) ~~ k[A][D]`.

---

`A stackrel(k_1" ")rightleftharpoons B + C` `" "" "`(`"fast equilibrium"`)
`""^(" "k_(-1))`
`ul(C + D stackrel(k_2" ")(->) E" "" ")` (`"slow"`)
`A + D stackrel(k" ")(->) B + E`

We begin with the notion that the slow step is the rate-determining step. From that, the initial form of the rate law is:

`r(t) = k_2[C][D]`

where `k_2` is the rate constant for the forward second step.

However, `C` is not a reactant (but is an intermediate), and as such, this is not an appropriate rate law yet. Instead, we should recognize that

`K = k_1/k_(-1) = ([B][C])/([A])`

is the equilibrium constant for the first reaction step. Therefore:

`[C] = k_1/k_(-1) ([A])/([B])`

and we can substitute back into the rate law to get:

`r(t) ~~ (k_2k_1)/(k_(-1)[B]) [A][D]`

We would use `[B]` at equilibrium, so the approximation is that `[B]` is a constant after step 1 and stays constant through step 2 in the reaction mechanism (because it isn't a reactant or product in step 2).

In the expected notation,

`color(blue)(r(t) ~~ k[A][D])`,

where `k = (k_2k_1)/(k_(-1)[B])`.