Question

What are the sine, cosine, and tangent of `theta = (3pi)/4` radians?

Answer 1

`sin((3pi)/4) = sqrt2/2`

`cos((3pi)/4) = -sqrt2/2`

`tan((3pi)/4) = -sqrt2/2`

Explanation:

first, you need to find the reference angle and then use the unit circle.

`theta = (3pi)/4`

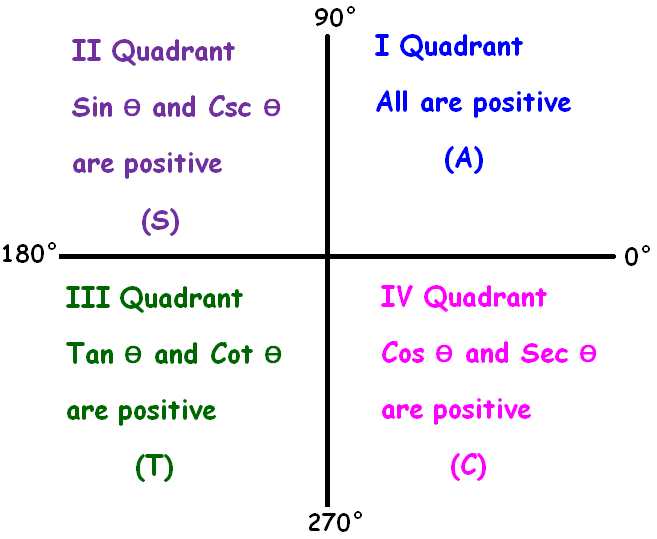
now to find the reference angle you have to determine that angle is in which quadrant

`(3pi)/4` is in the second quadrant because it is less than `pi`

which it is `(4pi)/4 = 180^@`

second quadrant means its reference angel = `pi - (3pi)/4 = pi/4`
then you can use the unit circle to find the exact values or you can use your hand!!

now we know that our angle is in the second quadrant and in the second quadrant just sine and cosecant are positive the rest are negative

---

enter link description here

---

so

`sin((3pi)/4) = sin(pi/4) = sqrt2/2`

`cos((3pi)/4) = -cos(pi/4) = -sqrt2/2`

`tan((3pi)/4) = -tan(pi/4) = -sqrt2/2`