Question

What is the change in the object’s velocity from t=4 to t=10?

The acceleration of an object at various times is shown in the table below. Using the information in the table, estimate the change in the object’s velocity from t=4 to t=10.

Answer 1

1. Assuming that acceleration changes from `t=4` to `t=6` linearly, we see that

   `dot a=(5.5-4.2)/2=0.65\ ms^-3`
   Integrating both sides with respect to time we get
   `a=0.65t+C` ......(1)
   where `C` is a constant of integration to be evaluated from initial conditions. It is given that at `t=4=>t_0=0, a=4.2`. Inserting in (1) we get
   `4.2=0.65xx0+C`
   `=>C=4.2`
   Inserting in (1) we get
   `a=0.65t+4.2` .......(2)
   We know that `a=dot v`. Integrating with respect to time both sides we get
   `v=int(0.65t+1.6)\ dt`
   `=>v=0.65t^2/2+4.2t+C_1` .....(3)
   where `C_1` is a constant of integration to be evaluated from initial conditions. Let us assume that at `t=4=>t_0=0, v=v_4`. Inserting in (3) we get
   `=>v_4=0.65xx0^2/2+4.2xx0+C_1`
   `=>C_1=v_4`
   Inserting in (3) we get
   `=>v=0.325t^2+4.2t+v_4`
   calculating velocity at `t=6=>` this acceleration was in operation for `2s`
   `v_6=0.325xx2^2+4.2xx2+v_4`
   `=>v_6=9.7+v_4` .........(4)

2. Assuming that acceleration changes from `t=6` to `t=8` linearly, we see that for this time interval

   `dot a=(5.1-5.5)/2=-0.2\ ms^-3`
   Integrating both sides with respect to time we get
   `a=-0.2t+C_2` ......(5)
   where `C_2` is a constant of integration to be evaluated from initial conditions. It is given that at `t=6=>t_0=0, a=5.5`. Inserting in (5) we get
   `5.5=-0.2xx0+C_2`
   `=>C_2=5.5`
   Inserting in (5) we get
   `a=-0.2t+5.5` .......(6)
   We know that `a=dot v`. Integrating with respect to time both sides we get
   `v=int(-0.2t+5.5)\ dt`
   `=>v=-0.1t^2+5.5t+C_3` .....(7)
   where `C_3` is a constant of integration to be evaluated from initial conditions. We have at `t=6=>t_0=0, v_6=9.6+v_4`. Inserting in (3) we get
   `=>9.6+v_4=-0.1xx0^2+5.5xx0+C_3`
   `=>C_3=9.6+v_4`
   Inserting in (7) we get
   `=>v=-0.1t^2+5.5t+9.6+v_4`
   calculating velocity at `t=8=>` this acceleration was in effect for `2s`
   `v_8=-0.1xx2^2+5.5xx2+9.6+v_4`
   `=>v_8=20.2+v_4` .........(8)

3. Assuming that acceleration changes from `t=8` to `t=10` linearly, we see that

   `dot a=(6.2-5.1)/2=0.55\ ms^-3`
   Integrating both sides with respect to time we get
   `a=0.55t+C_4` ......(9)
   where `C_4` is a constant of integration to be evaluated from initial conditions. It is given that at `t=8=>t_0=0, a=5.1`. Inserting in (9) we get
   `5.1=0.55xx0+C_4`
   `=>C_4=5.1`
   Inserting in (9) we get
   `a=0.55t+5.1` .......(10)
   We know that `a=dot v`. Integrating with respect to time both sides we get
   `v=int(0.55t+5.1)\ dt`
   `=>v=0.55t^2/2+5.1t+C_5` .....(11)
   where `C_5` is a constant of integration to be evaluated from initial conditions. We have calculated that at `t=8=>t_0=0, v_8=20.2+v_4`. Inserting in (11) we get
   `=>20.2+v_4=0.55xx0^2/2+5.1xx0+C_5`
   `=>C_5=20.2+v_4`
   Inserting in (11) we get
   `=>v=0.275t^2+5.1t+20.2+v_4`
   calculating velocity at `t=10=>` this acceleration was in operation for `2s`
   `v_10=0.275xx2^2+5.1xx2+20.4+v_4`
   `=>v_10=(31.7+v_4)\ ms^-1` .........(12)

Answer 2

`"10.6 m/s"`

Explanation:

Acceleration is given by

`"a" = "Δv"/"t"`

`"Δv = at"`

From above equation we can say that area of an acceleration vs time graph gives us change in velocity.

Acceleration vs Time graph from `4 - 10` seconds is shown below

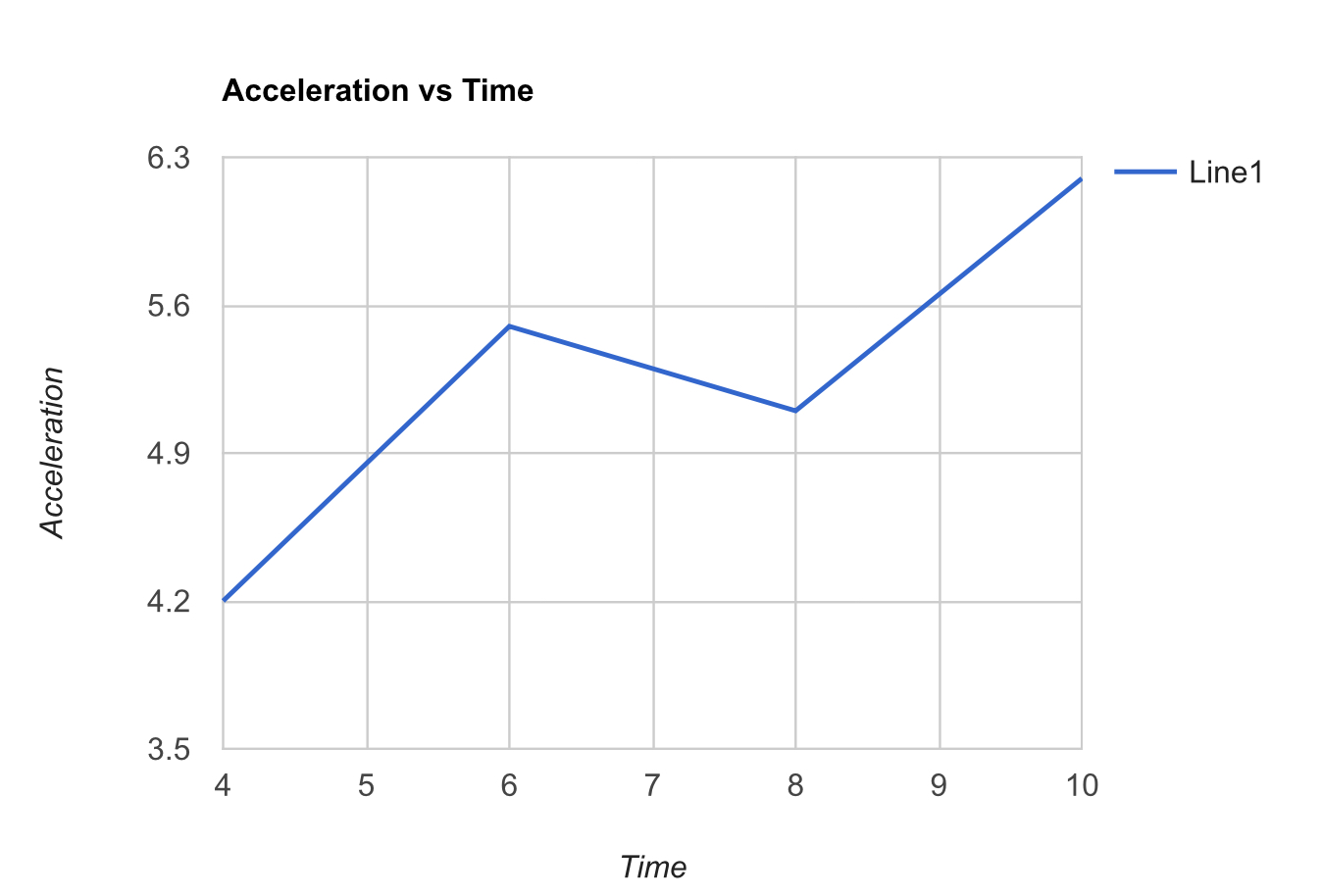
Area under this graph is change in velocity of object

1. From `t = 4` to `t =6`
   `"Δv"_1 = [1/2 × 2 × (5.5 - 4.2)] + [(6 - 4) × (4.2 - 3.5)]`
   `color(white)(Δv_1) = 1.3 + 1.4`
   `color(white)(Δv_1) = "2.7 m/s"`

2. From `t = 6` to `t = 8`
   `"Δv"_2 = [1/2 × 2 × (5.5 - 5.1)] + [(8 - 6) × (5.1 - 3.5)]`
   `color(white)(Δv_2) = 0.4 + 3.2`
   `color(white)(Δv_2) = "3.6 m/s"`

3. From `t = 8` to `t = 10`
   `"Δv"_3 = [1/2 × 2 × (6.2 - 5.1)] + [(10 - 8) × (5.1 - 3.5)]`
   `color(white)(Δv_3) = 1.1 + 3.2`
   `color(white)(Δv_3) = 4.3\ "m/s"`

Change in velocity from `t = 4` to `t = 10` is

`"Δv = Δv"_1 + "Δv"_2 + "Δv"_3`

`color(white)(Δv) = "2.7 m/s + 3.6 m/s + 4.3 m/s"`

`color(white)(Δv) = "10.6 m/s"`