A) In circle P, find the length of AB given that the radius r = 7 inches and m APB 4 radians?

Question

1201 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-06-05T17:54:03

#12.73#

We want to begin this with a diagram.

Assuming that P is the origin of the circle, we have the following scenario:

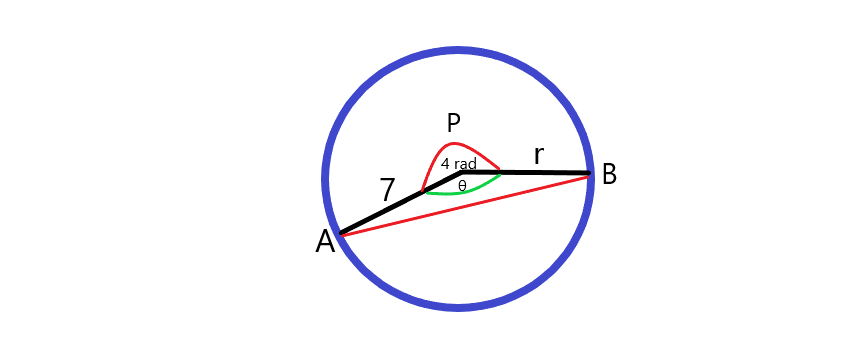
enter image source here

#r=7#

The length AB is the longest side of an isosceles triangle with other side lengths #7#. The angle between them on the diagram is #theta#.

We are told that the angle of APB is #4#. The angle of our triangle, #theta=2pi-4#

From here, we can use the cosine rule.

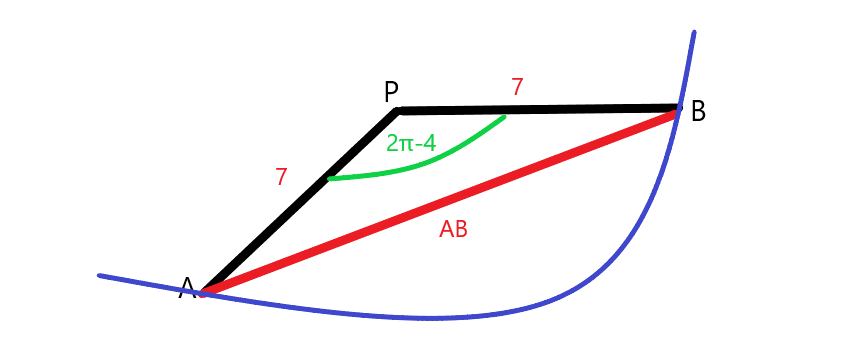Zoomed in version of just the triangle

#AB^2=7^2+7^2-2*7*7cos(2pi-4)#

#AB^2=98-98cos(2pi-4)#
#AB^2=162.06#
#AB=12.73#