What is the equation in standard form of the parabola with a focus at (21,15) and a directrix of y= -6?

Question

1248 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-06-15T07:09:29

#(x-21)^2=42(y-4.5)#

Given -

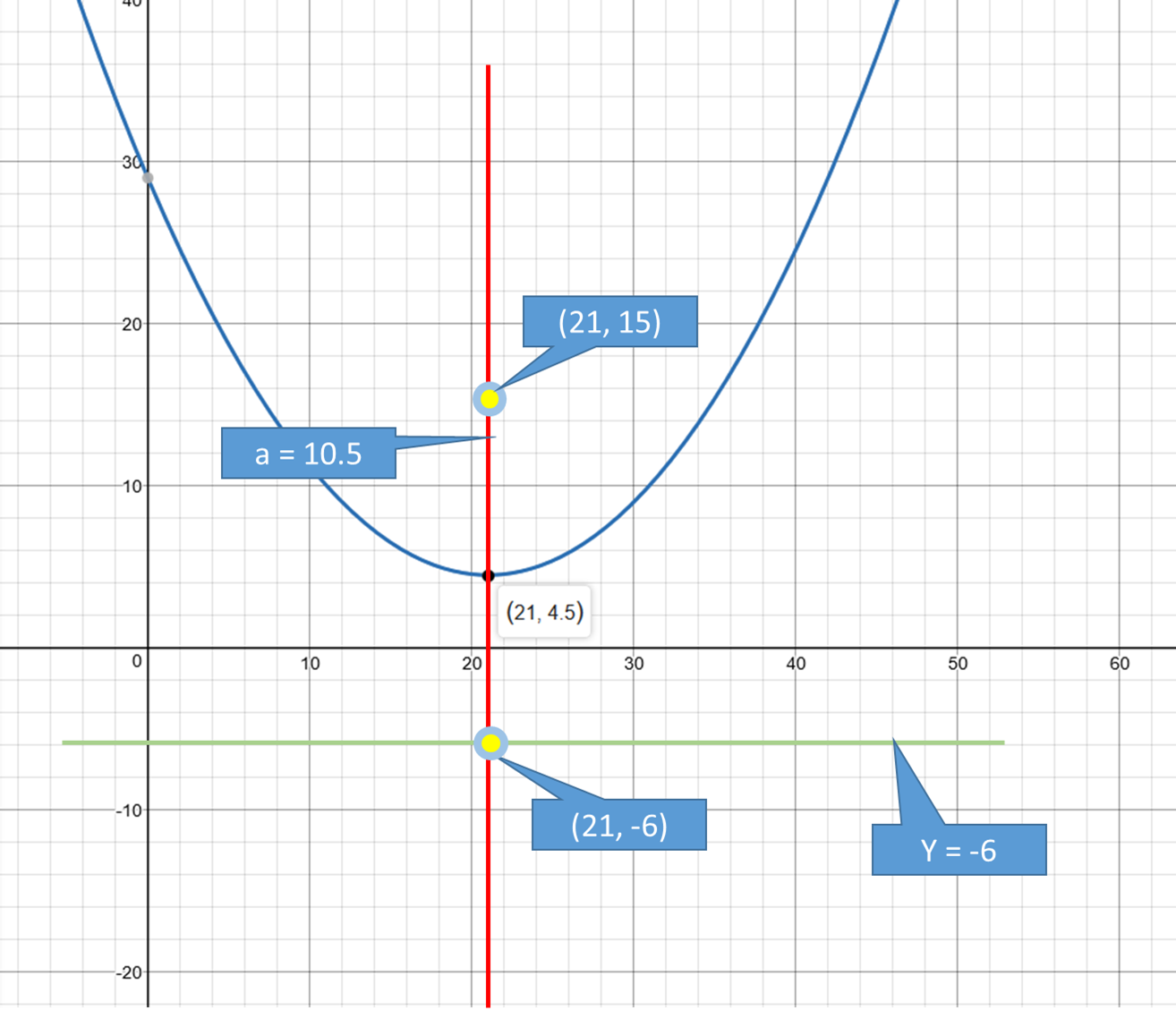
Focus #(21, 15)#
Directrix #y=-6#

This parabola opens up. Its origin is away from the origin #(h, k)#.

Where -

#h=21#
#k=4.5#
#a=10.5#
Look at the graph

enter image source here

Hence the general form of the equation is -

#(x-h)^2 = (4)(a)(x-k)#

#x-21)^2=(4)(10.5)(y-4.5)#

#(x-21)^2=42(y-4.5)#