Question

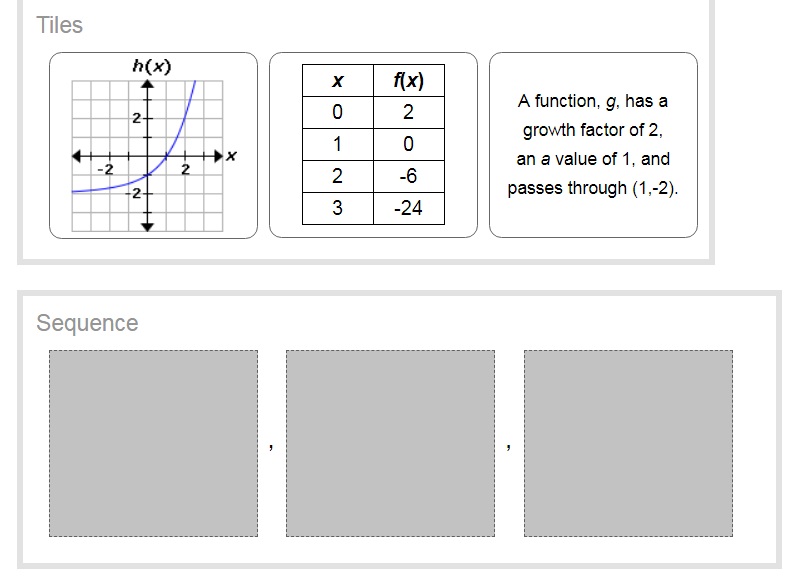
Find the y-intercept of each exponential function and order the functions from least to greatest y-intercept. ?

Answer 1

Tile 1 - Tile 3 - Tile 2

or

Tile 3 - Tile 1 - Tile 2

Explanation:

Tile One:

The `y`-intercept is `-1` as shown in the graph.

Tile Two:

The coordinates of a `y`-intercept always has its `x`-coordinate as `0`. i.e. `(0, y)`

Thus, in the table, the `y`-intercept can be found by finding the value of `y` (which is equivalent to `f(x)` ) when `x = 0` which is `f(x) = 2`.

Tile Three:

If we put the explanation into a function, it would become:

`g(x) = a\times2^x` where a is an unknown constant.

If we inserted the coordinates into the function that we've created, we get:

`-2 = 2a`

`\therefore a = -1`

and now, we can complete the function to get:

`g(x) = -2^x`

To find the `y`-intercept, we have the `x`-coordinates as `0`:

`g(0) = -2^0`

`g(0) = -1`

`\thereforey`-intercept is `-1`.

Final answer:

Now we can just arrange the tiles in order of the values of the `y`-intercept.

Thus, the order is:

Tile 1 - Tile 3 - Tile 2

or

Tile 3 - Tile 1 - Tile 2

Hope that makes sense!