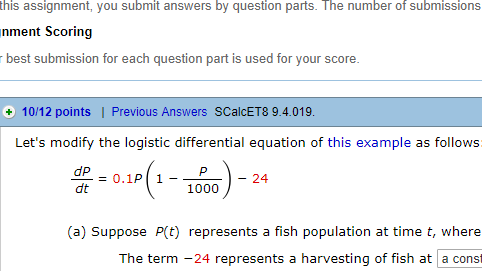
Solve this differential equation explicitly, either by using partial fractions, or with a computer algebra system. Use the initial populations 350 and 450. Can anyone please help me with this problem and explain how you got the answers?

Question

4178 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-06-24T08:39:32

For the IV: #P_o = 350#

For the IV: #P_o = 450#

#dot P = P/10 - P^2/10000 - 24 #

Complete square:

#= 1 - (P - 500)^2/10000#

Let #Q = P - 500 qquad dot Q = dot P#

So the DE is:

#dot Q = 1 - (Q/100)^2#

Let #R = Q/100 qquad dot R = (dotQ)/100#

#100 dot R = 1 - R^2#

This separates:

#(dR)/(1 - R^2) = 1/100dt#

Factor and use partial fractions:

#= int dR \ 1/((1 - R)(1+R)) = 1/100 int \dt#

#=1/2 int dR \ 1/ (R + 1) - 1/ (R - 1) = t/100 + C#

#=1/2( ln (R + 1) - ln (R - 1) ) = t/100 + C#

#= ln ((R + 1)/ (R - 1) ) = t/50 + C#

#R = (Ce^( t/50) + 1)/(Ce^( t/50) - 1)#

Reverse the subs:

# Q= 100 (Ce^( t/50) + 1)/(Ce^( t/50) - 1)#

#bb( P= 100 ((Ce^( t/50) + 1)/(Ce^( t/50) - 1) + 5 )#

For the IV: #P_o = 350#

#350 = 100 ((C + 1)/(C - 1) + 5 ) implies C = 1/5#

# P= 100 ((e^( t/50) + 5)/(e^( t/50) - 5) + 5 )#

For the IV: #P_o = 450#

#450 = 100 ((C + 1)/(C - 1) + 5 ) implies C = -1/3#

# P= 100 (( e^( t/50) - 3)/( e^( t/50) + 3 ) + 5 )#