Question

On average, how many `pi` bonds does `"O"_2^(+)` have?

Answer 1

As mo theory there should be 1.5 pi bond

Explanation:

MO configuration of `O_2^+`

Answer 2

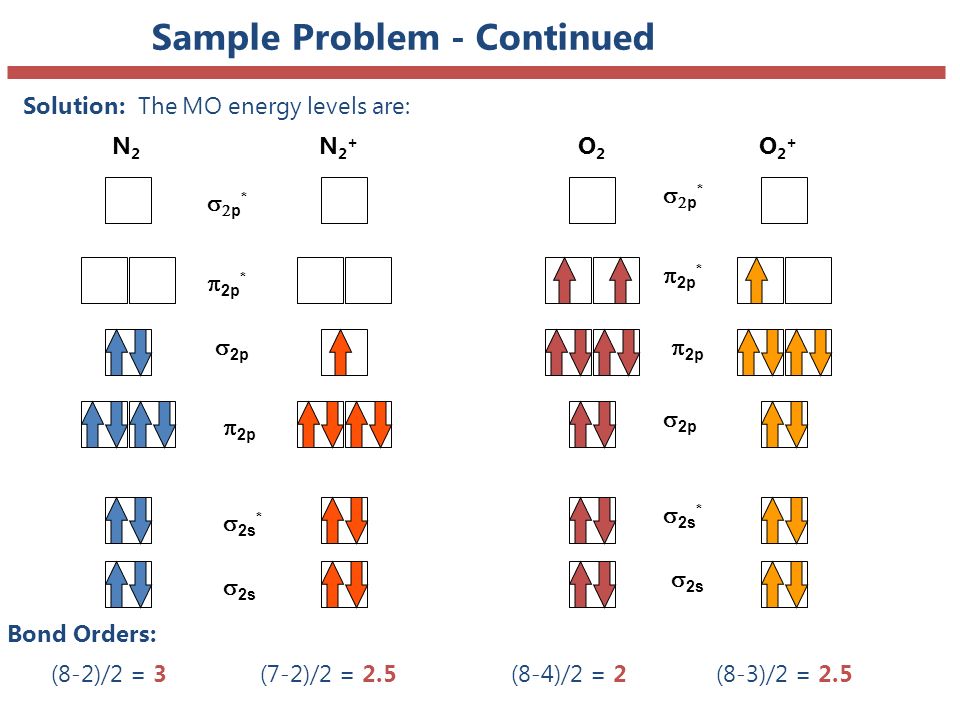
You would have a bond order of `2.5` in `"O"_2^(+)`. Remembering that `"O"_2^(+)` has one `sigma` bond as well, you therefore have `1.5` `pi` bonds on average.

---

BOND ORDER OF HOMONUCLEAR DIATOMICS

Bond order is a measure of bond strength, and suggests stability. It is half the number of bonding minus the number of antibonding molecular orbitals.

`"Bond Order" = ("Bonding e"^(-) - "Antibonding e"^(-))/2`

`pi` bonds are made when all lobes of an orbital overlap in parallel (such as two `2p_x` or two `d_(xz)` orbitals, where the `x` axis is toward you and the `z` axis is upwards).

You can have optimal overlap, less than optimal overlap, or no overlap. Poorer bonding overlaps correspond with lower values of bond order. Or, less antibonding overlap corresponds with higher values of bond order (which is what applies here).

The structures of `"O"_2` and `"O"_2^(+)` are:

DIATOMIC OXYGEN IS PARAMAGNETIC

While Valence Bond Theory suggests oxygen is diamagnetic, Molecular Orbital Theory correctly demonstrates that oxygen, `"O"_2`, is paramagnetic.

That means it has unpaired electrons. Specifically, two unpaired electrons, one in each `pi` antibonding orbital (`pi_(2px)^"*"` and `pi_(2py)^"*"`), where the `z` direction is along the internuclear axis.

The MO diagram for neutral `"O"_2` is:

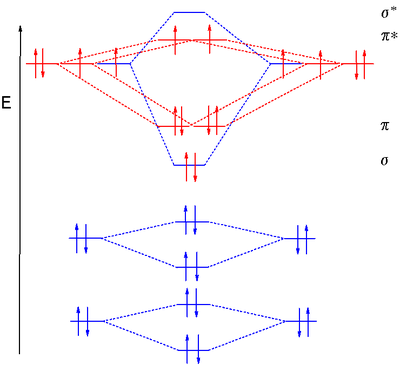
When you take away one electron, you take it away from the highest-occupied molecular orbital. Since either the `pi_(2px)^"*"` or `pi_(2py)^"*"` can function as such (they are the same energies), one of these orbitals can lose an electron when we wish to form `"O"_2^(+)`.

DETERMINING BOND ORDER

Naturally, `"O"_2` has a bond order of `2` which corresponds nicely with its double bonded Lewis structure.

Two bonding electrons each come from the `sigma_(1s)`, `sigma_(2s)`, `sigma_(2pz)`, `pi_(2px)`, and `pi_(2py)` molecular orbitals for a total of `10`. Two antibonding electrons each come from the `sigma_(1s)^"*"` and `sigma_(2s)^"*"`, and one each from the `pi_(2px)^"*"` and `p_(2py)^"*"` molecular orbitals for a total of `6`.

`(10 - color(red)(6))/2 = color(blue)(2)`

When taking away one `pi^"*"` antibonding electron to form `"O"_2^(+)`, we change the bond order to:

`(10 - color(red)(5))/2 = color(blue)(2.5)`

Since `"O"_2^(+)` has lost one of two antibonding `pi` electrons, its bonds get less weak by half. So, instead of going from `1` `pi` bond to `0.5` `pi` bonds, it goes to `\mathbf(1.5)` `\mathbf(pi)` bonds.