Question

Why is the area of a circle given by the formula `pir^2` ?

Answer 1

See explanation...

Explanation:

The circumference of a circle is `pi` times its diameter. In fact that's the original definition of `pi`. SInce the diameter is twice the radius, that means that the circumference of a circle of radius `r` is `2pir`.

If we take a circle of radius `r` and cut it into a number of segments, we can then reassemble those segments into a sort of bumpy parallelogram with height `r` and longer sides of length `pir` (each being half the circumference).

If we use a larger number of thin segments, then this is more like a rectangle with height `r` and base `pir`, which therefore has area `r*pir = pir^2`

Here's an animation for just `8` segments...

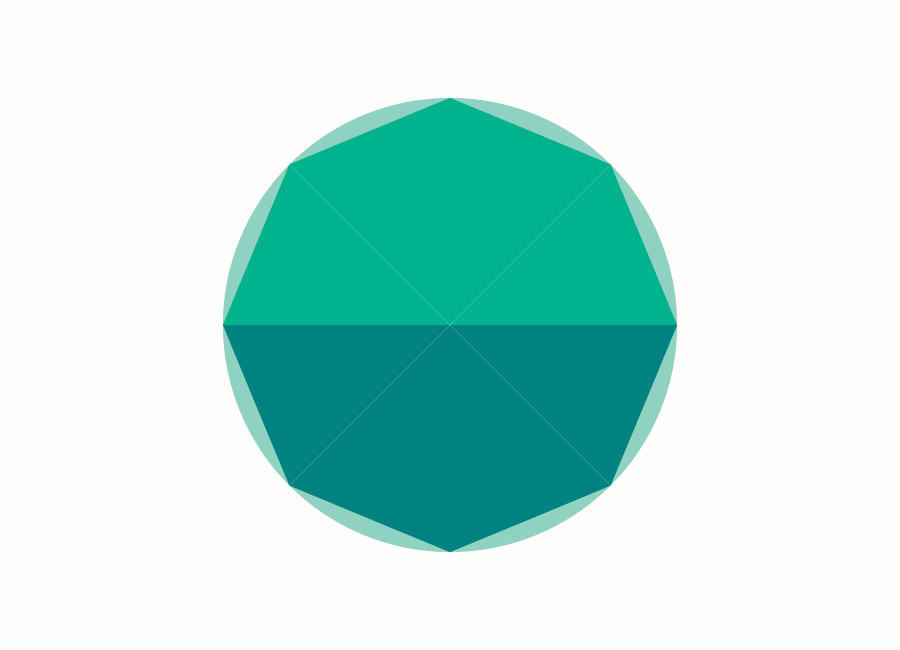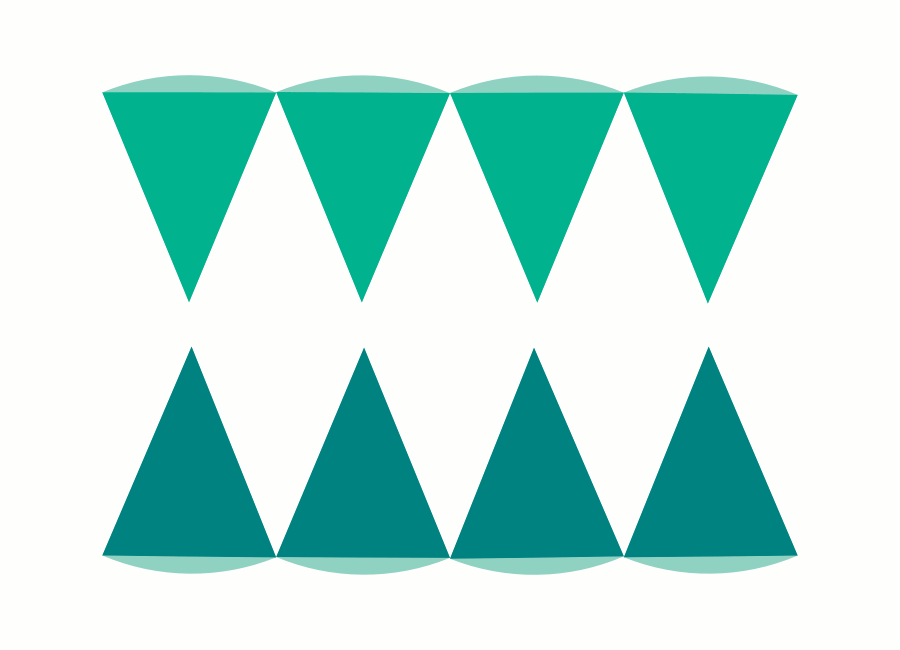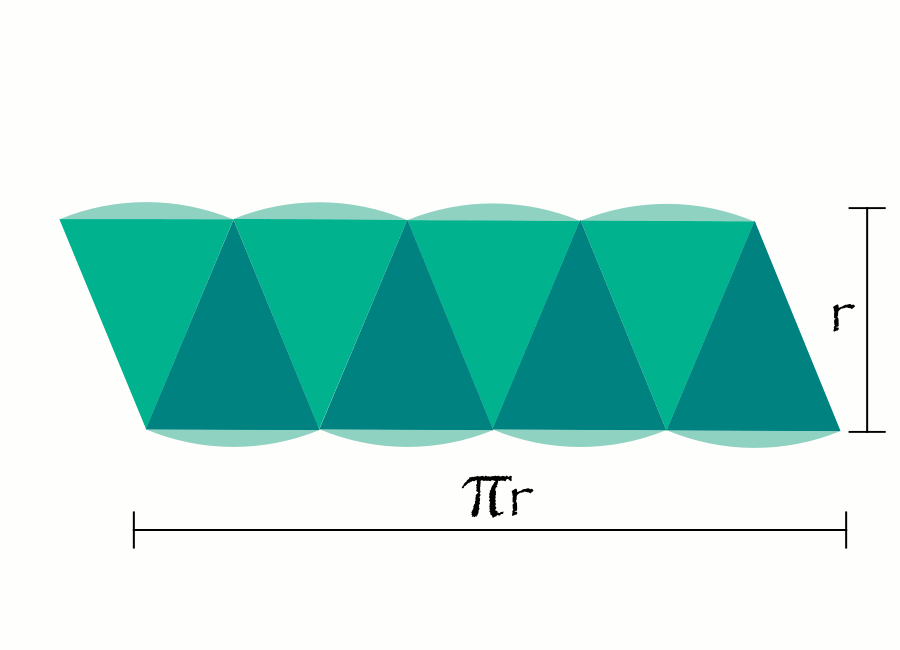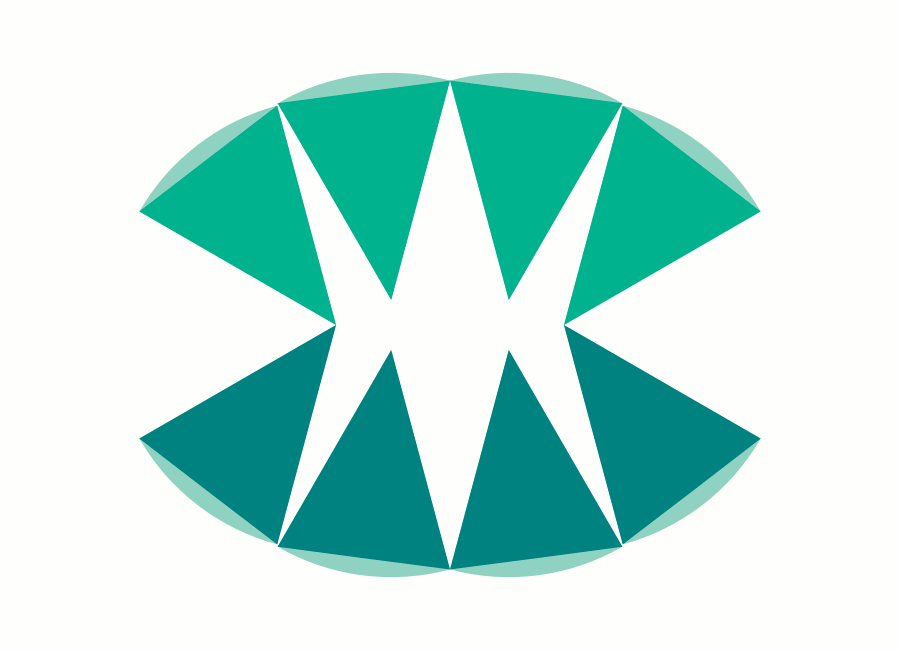
So the area of the circle is proportional to the square of the radius.