Question #c20a4

Question

2465 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-04-04T23:31:32

I tried this:

Have a look:
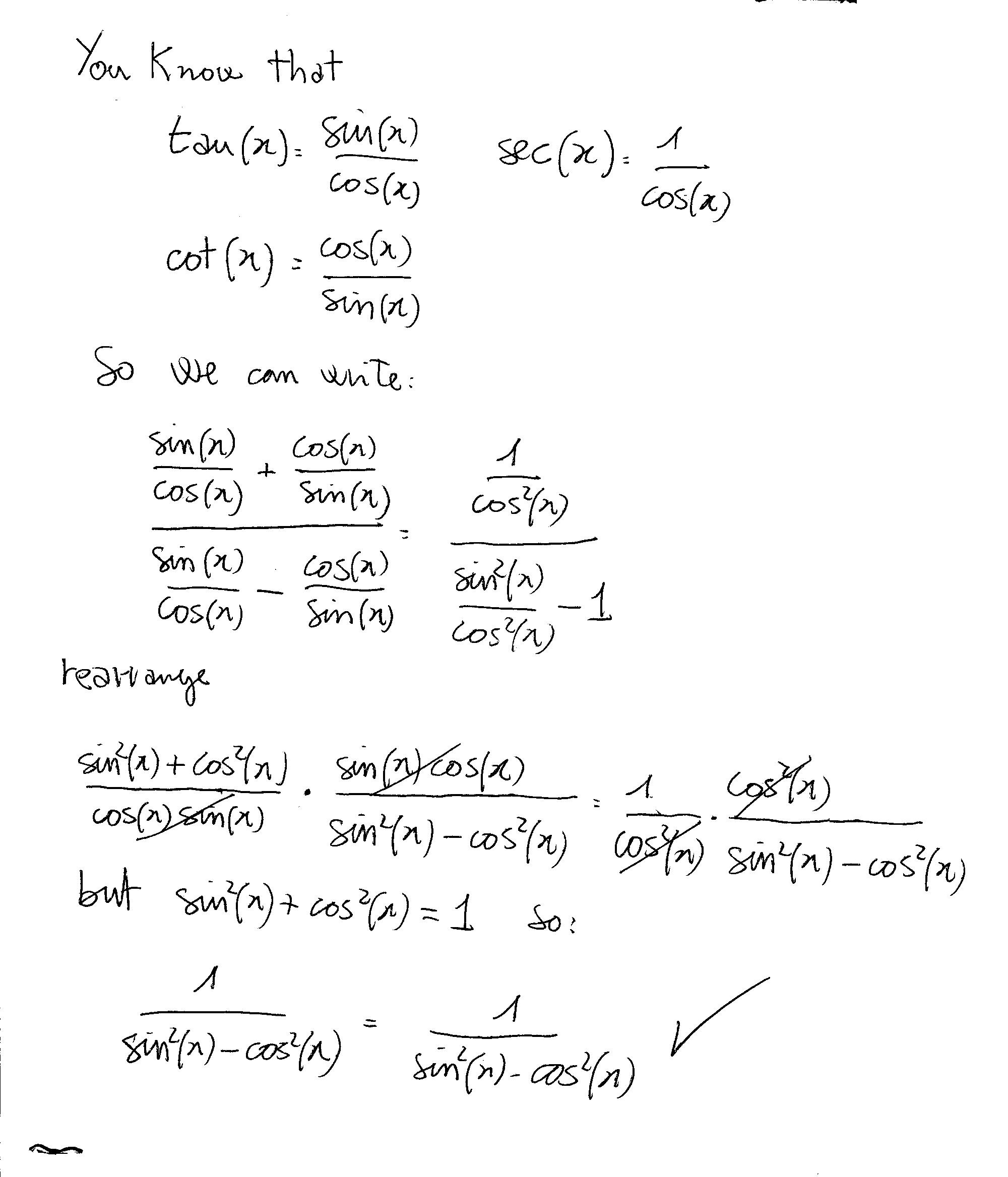
enter image source here

Answer 2 · 2017-04-04T23:32:25

#(tanx+cotx)/(tanx-cotx)=sec^2x/(tan^2x-1)#

Multiply the left-hand side by the conjugate of the denominator:

#(tanx+cotx)/(tanx-cotx)=(tanx+cotx)/(tanx-cotx)*(tanx+cotx)/(tanx+cotx)#

#=(tan^2x+2tanxcotx+cot^2x)/(tan^2x-cot^2x)#

Rewriting #cotx#:

#=(tan^2x+2tanx(1/tanx)+1/tan^2x)/(tan^2x-1/tan^2x)#

Multiplying through by #tan^2x#:

#=(tan^4x+2tan^2x+1)/(tan^4x-1)#

Factoring:

#=(tan^2x+1)^2/((tan^2x+1)(tan^2x-1))=(tan^2x+1)/(tan^2x-1)#

The numerator is a form of the Pythagorean identity:

#=sec^2x/(tan^2x-1)#

This is the right-hand side of the original equation, so the identity has been verified.