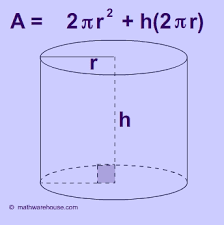
General equation for the Total Surface Area of a cylinder is
A = 2 pi r^2 + 2 pi r h
r^2 + rh = A / (2 pi)
r^2 + h r - (A / (2 pi)) = 0
Formula to find roots of a quadratic equation
ax^2 + b x c = 0 is given by
x = (-b +- sqrt(b^2 + 4 a c)) / (2a)
:. r = ( - h +- sqrt(h^2 + ((4A)/( 2 pi)))) / 2
r = (-h +- sqrt(h^2 + ((2A) / (pi)))) / 2
In this problem,
A = 1350, h = 10 d = 20r
:.1350 = 2pir^2 + 2pirh = 2pi(r^2 + r * 20r)
2 pir^2 (1 + 20) = 1350
r^2 = 1350 / (2pi * 21)
r^2 = 1350 / ((2 * 22 * cancel(color (red)(21) )3 )/cancel(color(red)(7))) = 1350 / 132
r = sqrt(1350/132) = 3.198 cm


