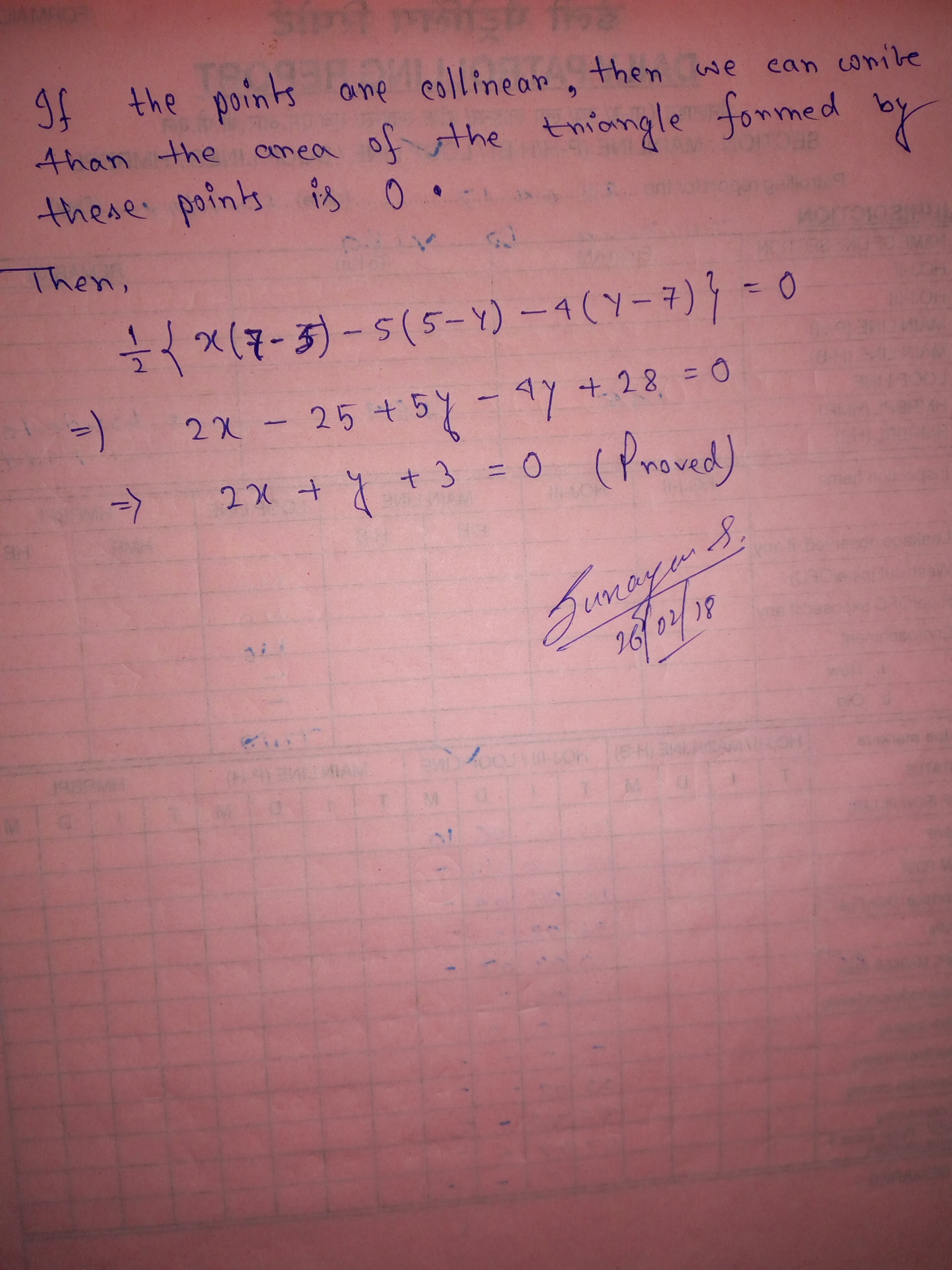What is the condition that makes three points #(x,y)#, #(-5,7)# and #(-4,5)# collinear?

Question

972 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-02-26T16:44:49

Please see below.

If the three points #(x,y)#, #(-5,7)# and #(-4,5)# are collinear, then the slope between any two points should be equal.

Slope between #(x,y)# and #(-5,7)# is #(y-7)/(x+5)#

snd slope between #(-5,7)# and #(-4,5)# is #(5-7)/(-4-(-5))=(-2)/1=-2#

Hence we have #(y-7)/(x+5)=-2#

or #y-7=-2x-10#

or #2x+y+3=0#

Note #-# This is the equation of line joining points #(-5,7)# and #(-4,5)#.

Answer 2 · 2018-02-26T16:46:16

See the answer below...

My notebook...