Question #ba71a

1 Answer
Sridhar V.
Feb 8, 2018

Rolle's Theorem is satisfied as there are two points at

#color(blue)(x=1.868517," " x = -0.53518#

where #color(green)(f'(x) = 0#

Explanation:

Rolle's Theorem states that under certain conditions an extreme value is guaranteed to lie in the interior of the closed interval.

Rolle's Theorem

Conditions:

  1. The function #color(blue)(f(x)# must be continuous on the closed interval [a, b].

  2. The function f(x) must be differentiable on the open interval (a, b).

  3. f(a) = f(b)

If these three conditions are satisfied then there is at least one number in the open interval (a, b) such that the derivative of f(x) is zero.

Given:

#color(brown)(f(x) = x^3-2x^2-3x#

Observe that #color(brown)(f(x) = x^3-2x^2-3x# is a Cubic Function.

We will now find the x-intercepts of #f(x)#

#color(green)(f(x) = x^3-2x^2-3x#

and show that

#f'(x) = 0 # at some points between the x-intercepts

We have

#f(x) = x^3-2x^2-3x#

#f(x) = x(x^2-2x-3)#

We can write this expression as

#f(x) = x(x^2+x-3x-3)#

#f(x) = x[x(x+1)-3(x+1)]#

#rArr x*(x+1)*(x-3)#

Hence the solutions to our Cubic Function are

#color(blue)(x=0; x= (-1); x= 3#

Please refer to the image of the graph below that visually presents our findings.

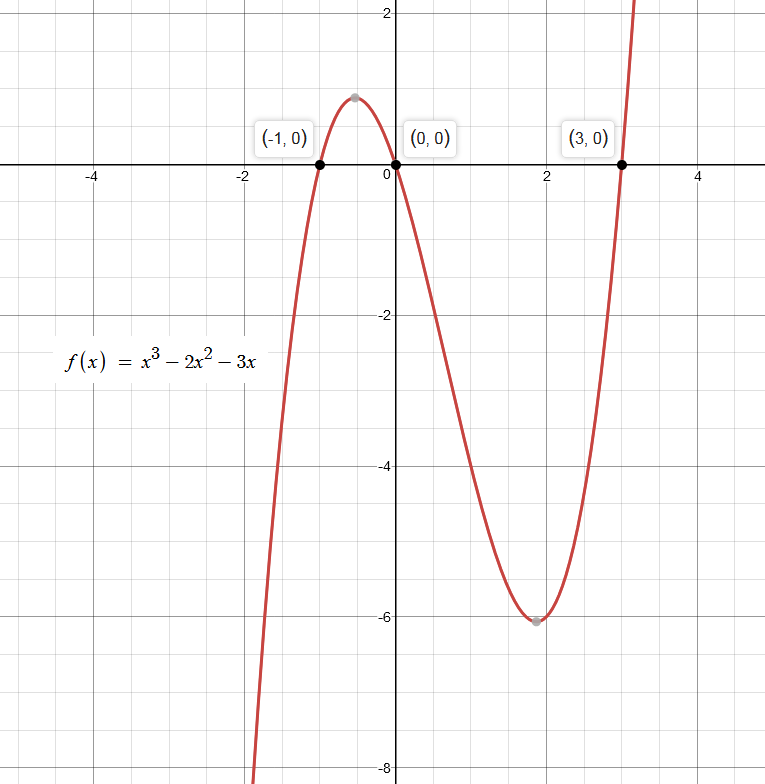
enter image source here

Next

we will differentiate #color(green)(f(x) = x^3-2x^2-3x#

We will find

#d/dx [x^3 - 2x^2 - 3x]#

#rArr 3x^2-4x-3#

#:. f'(x) = 3x^2-4x-3#

Next

we will set #color(brown)(f'(x) = 0#

i.e., #3x^2-4x-3 = 0#

This is a quadratic equation

General Form of a quadratic equation is

#color(red)(ax^2+bx+c=0#

Consider our quadratic equation

#3x^2-4x-3 = 0#

Note that

#color(blue)(a=3; b=(-4) and c=(-3)#

We will use the following quadratic formula to simplify:

#x=[ -b +- sqrt(b^2 - 4ac)]/(2a)#

#x=[ -(-4) +- sqrt((-4)^2 - 4*3*(-3))]/(2*3)#

#x=[4+-sqrt(52)]/6#

Hence our roots are

#x=(2/3)+(1/3)*sqrt(13) " "(or)" "#

#x = (2/3)+(-1/3)*sqrt(13)#

#color(blue)(rArr x = 1.868517 or x = - 0.53518#

Rolle's Theorem is satisfied as there are two points at

#color(blue)(x=1.868517," " x = -0.53518#

where #color(green)(f'(x) = 0#

Please refer to the image of the graph below that visually presents our findings.

enter image source here

Related questions
Impact of this question
361 views around the world
You can reuse this answer
Creative Commons License