Question

A block weighing `8 kg` is on a plane with an incline of `pi/4` and friction coefficient of `2`. How much force, if any, is necessary to keep the block from sliding down?

Answer 1

`0 \ N`, the object would remain at rest

Explanation:

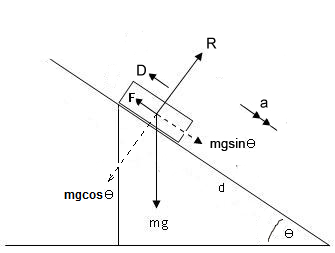
The diagram shows the forces acting on the block, and we have introduced the following variables:

# { (D, "Driving Force to stop block from sliding", N), (F, "Frictional force", N), (R, "Reaction Force from Slope (Newton's 3rd Law)", N) , (m, "Mass of object", 8 \ kg), (a, "acceleration of object down the slope", ms^-2), (theta, "Angle of the slope", pi/4 \ "radians"), (g, "gravitational constant", 9.8 \ ms^-2), (mu, "Static coefficient of friction for block", 2) :} #

We would expect the force from gravity to overcome the force of friction and for the block to slide down the plane, However if this is not the case then the maths below will show that this by requiring that `D le 0`

Applying Newton's 2nd Law Perpendicular to the plane we get:

`R-mgcostheta = 0`
`:. R=8gcos(pi/4)` .......... [1]

We want the block to be in equilibrium with the force `D` sufficient to stop the block from sliding, so we require no downwards acceleration `=>a=0`, Applying Newtons's 2nd Law Parallel to the plane downwards we get:

`mgsin theta -F -D = 0`
`:. 8gsin(pi/4) - F - D = 0` .......... [2]

If the block is just about to slip we have limiting friction so:

`\ \ \ \ \ F=muR`
`:. F=2*8gcos(pi/4)` (from [1])
`:. F=16gcos(pi/4)` (from [1])

Substituting back into [2] we get:

`8gsin(pi/4) - 16gcos(pi/4) - D = 0`
`D= 8gsin(pi/4) - 16gcos(pi/4)`

and taking `9=9.8` we get:

`\ \ \ \ \ D=-55.43717 ... N`
`:. D=-55.44 \ N` (2dp)

Because `D` is negative the mathematics is indicating that we could apply a force of up-to `55.44 \ N` down the slope before the object would even start to move, ie no further force is required to stop the object from sliding (due mainly to the friction coefficient).