A charge of #2 C# is at the origin. How much energy would be applied to or released from a # 3 C# charge if it is moved from # ( -4, 6 ) # to #( 7 , -4 ) #?

Question

1650 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-06-05T15:24:35

#=-0.7895 # #GJ#

The potential energy can be given by:

#V(r) = (Q_1Q_2)/(4pi epsilon_0r)#

Where #Q# are the magnitudes of the charges and #r# is the distance between the charges.

We already know that the charges (which are constant) are:

#Q_1=2C# and #Q_2=3C# so we can plug these in to the top (ignoring units for now) to get:

#V(r)=(6)/(4pi epsilon_0r)=(3)/(2pi epsilon_0 r)#.

We wish to see the energy difference in moving the #3C# charge from #(-4,6)# to #(7,-4)#.

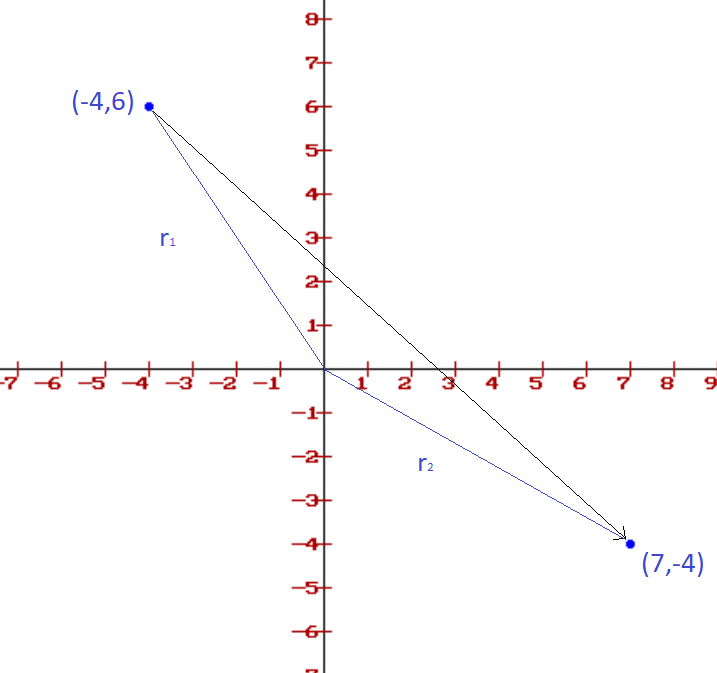
So we need to find #r# for both these cases (the scalar distance from the origin) which can be done by simple Pythagoras.

#r_1=sqrt((-4)^2+6^2)~~7.2111m#
#r_2=sqrt(7^2+(-4)^2)~~8.0623m#

assuming the coordinates given are in metres.

As it is a repulsive force (same sign charges) and we are moving further away then energy is being released.

To calculate how much we simply need:

#DeltaV=V(r_2)-V(r_1)#
#=(3)/(2pi epsilon_0times 8.0623)-(3)/(2pi epsilon_0times 7.2111)#

#=6.6887times10^9-7.4783times10^9#

#=-7.895times10^8J#

#=-0.7895 # #GJ#