Question

A composite geometric shape of triangle and rectangle is given with the proportionate relation given in the figure. Find angle `alpha` and `theta`?

Answer 1

`theta = arctan(2/3) ~~ 33.69^@`

`alpha= 90^@ - arctan(1/3)-arctan(1/4)~~57.53^@`

Explanation:

Labeling the picture as follows (with the additional constructed segment `bar(AE)`):

---

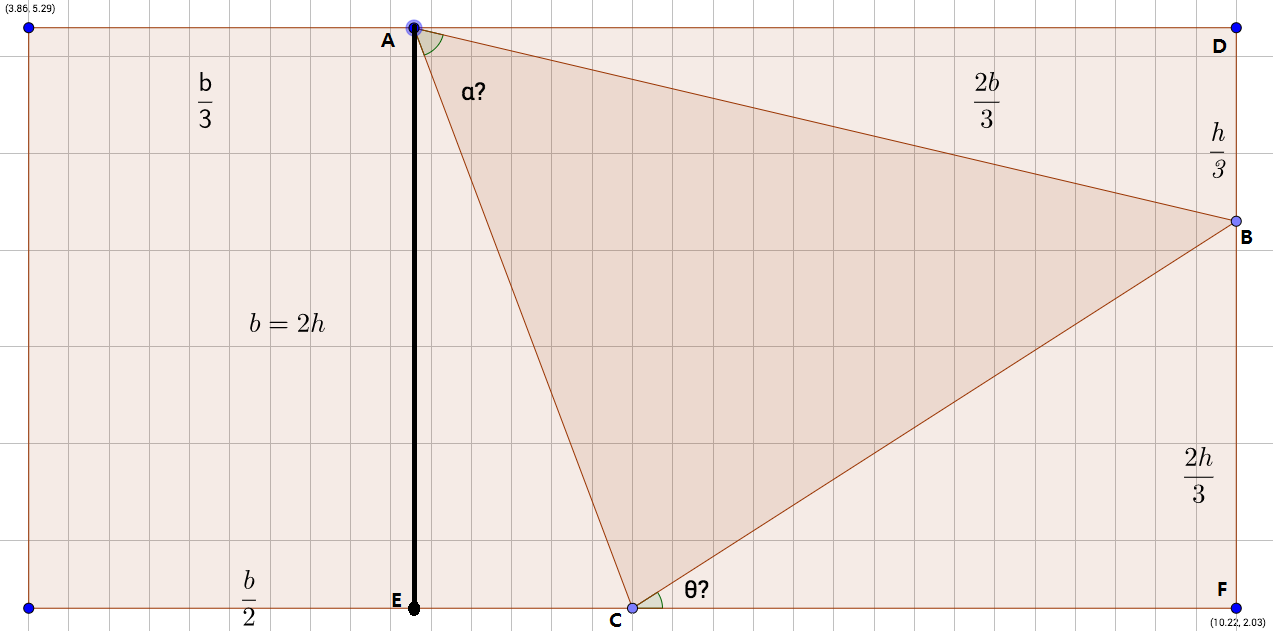
From the right triangle `triangleBCF`, given `bar(CF)=b/2=h` and `bar(BF) = (2h)/3`, we have `tan(theta) = ((2h)/3)/h=2/3`

Applying the inverse tangent function gives us `theta`:

`theta = arctan(2/3)~~33.69^@`

---

Note that `bar(EC) = bar(EF)-bar(CF) = bar(AD)-bar(CF)=(2b)/3-b/2 = b/6 = h/3`

Together with `bar(AE) = h`, we have the angle `angleEAC = arctan((h/3)/h) = arctan(1/3)`

Additionally, as `bar(BD) = h/3` and `bar(AD) = (2b)/3 = (4h)/3`, we have the angle `angleDAB = arctan((h/3)/((4h)/3)) = arctan(1/4)`

Noting that `angleEAD` is a right angle, we can now obtain `alpha`:

`alpha = angleEAD - angleEAC - angleDAB`

`= 90^@ - arctan(1/3)-arctan(1/4)~~57.53^@`