Question

Consider the following sets of quantum numbers. Circle all of the allowed sets of quantum numbers for an electron in a hydrogen atom. The electron may be either in a ground state or an excited state?

a) n=2, l=1, ml =-1, ms=+1/2

b) n=2, l=1, ml =2, ms=+1/2

c) n=2, l=2, ml =1, ms=+1/2

d) n=3, l=0, ml =0, ms=-1/2

e) n=3, l=1, ml =0, ms=0

f) n=0, l=0, ml =0, ms=-1/2

Answer 1

Here's what I got.

Explanation:

All you have to do here is compare the values given to your for the principal, angular momentum, magnetic, and spin quantum numbers with the allowed values they can take in relation to each other.

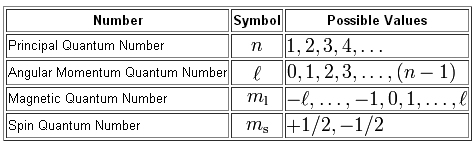
You will have

• (a) `" "n=2, l=1, m_l = 1, m_s = +1/2" "color(green)(sqrt())`

This set is valid because all four quantum numbers have allowed values. The angular momentum quantum number can take values ranging from `0` to `n-1`, so for `n=2` you will have

`l=0" "` and `" "color(darkgreen)(ul(color(black)(l=1)))`

The magnetic quantum number can take values ranging from `-l` to `+l`, so for `l=1` you have

`m_l = {-1, color(white)(-)0, color(darkgreen)(ul(color(black)(+1)))}`

Finally, the spin quantum number can take the value `+1/2`, so this represents a valid set of quantum numbers.

• (b) `" "n=2, l=1, m_l = 2, m_s = +1/2" "color(red)(xx)`

This set is not valid because the magnetic quantum number has a value that is not allowed for `l=1`, i.e. `m_l = +2`.

• (c) `" "n=2, l=2, m_l = +1, m_s = +1/2" "color(red)(xx)`

This set is not valid because the angular momentum quantum number cannot take the value `l=2` for `n=2`.

• (d) `" "n=3, l=0, m_l = 0, m_s = -1/2" "color(green)(sqrt())`

This set is valid because all four quantum numbers have allowed values. The angular momentum quantum number can take the value `l=0` when `n=3`,

Likewise, the magnetic quantum number can take the value `m_l = 0` when `l=0`. In fact, `m_l = 0` is the only allowed value for the magnetic quantum number when `l=0`.

• (e) `" "n=3, l=1, m_l = 0, m_s = 0" "color(red)(xx)`

This set is not valid because the spin quantum number can only take two values, `m_s = -1/2` or `m_s = +1/2`.

• (f) `" "n=0, l=0, m_l = 0, m_s = -1/2" "color(red)(xx)`

This set is not valid because the principal quantum number cannot take the value `n=0`.