Question

'Find the equation of the tangent to the curve ay^2=x^3 at the point (at^2,at^3) where a>0 and t is a parameter ?

Answer 1

`y = 3/2tx - 1/2at^3`

Explanation:

We have a family of curves defined for `a gt 0` by:

`C(a) : " " ay^2 = x^3` ..... [A]

Or in parametric form:

`C(a) : " " { (x(t)=at^2), (y(t)=at^3) :}`

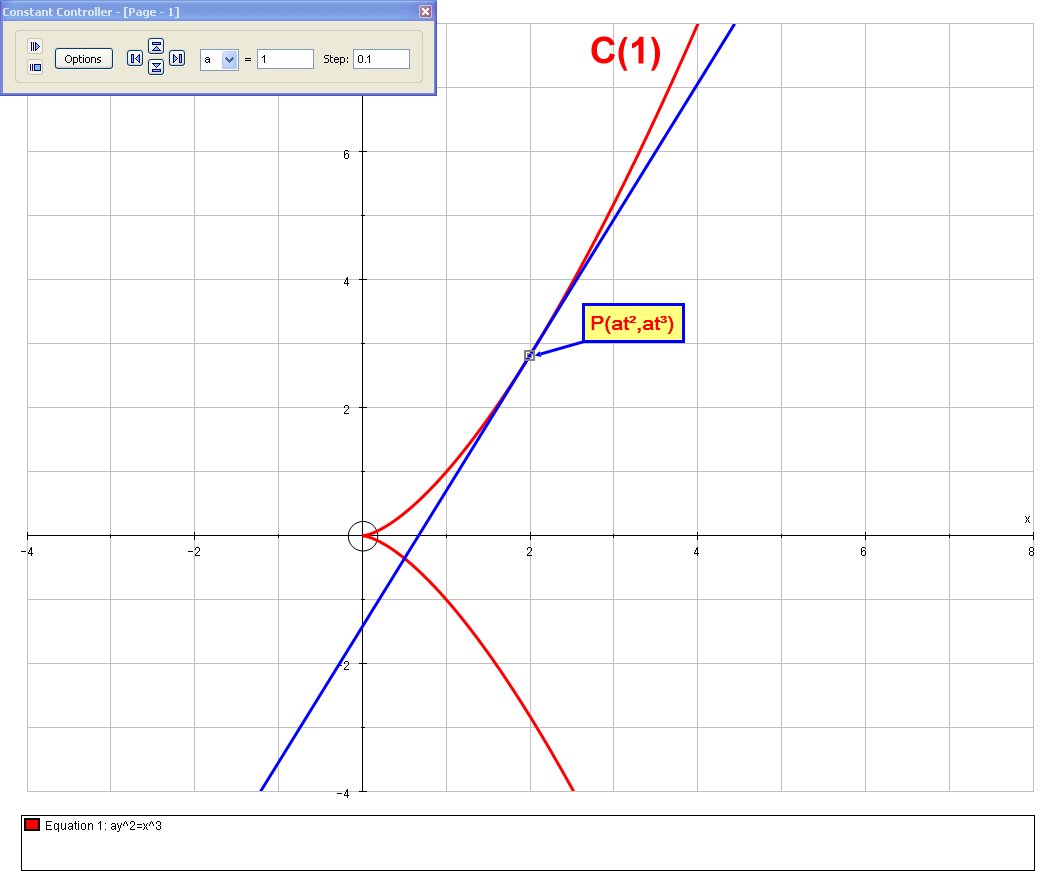
Here we have a plot for `a=1`

If we differentiate equation [A] implicitly wrt `x`, we have:

`2ay dy/dx = 3x^2 => dy/dx = (3x^2)/(2ay)`

The gradient of the tangent to a curve at any particular point is given by the derivative of the curve at that point. So, at a generic point `P(at^2,at^3)`, the derivative is:

`m_T = (3x^2)/(2ay) = (3(at^2)^2)/(2a(at^3)) = (3a^2t^4)/(2a^2t^3) = 3/2t`

So the tangent passes through `P(at^2,at^3)` and has gradient `m_T=3/2t`, and using the point/slope form `y-y_1=m(x-x_1)` the tangent equation we seek is;

`y - at^3 = 3/2t( x - at^2 )`

`:. y - at^3 = 3/2tx - 3/2at^3`

`:. y = 3/2tx - 1/2at^3`

Which is the sought equation.