Find the net torque and angular acceleration of the bar?
The drawing shows a bar lying on the surface of a horizontal table top. The bar is pivoted at the left end and is free to rotate with negligible friction in the horizontal plane of the table-top about the axis through the end. The bar is 2.0m long and has a mass of 0.60 kg.
The forces are as follows:
F1 = 14.0 N (at the pivot)
F2 = 12.0 N (at the 1.25m mark perpendicular to the bar)
F3 = 14.0 N (at the 2.0m mark)
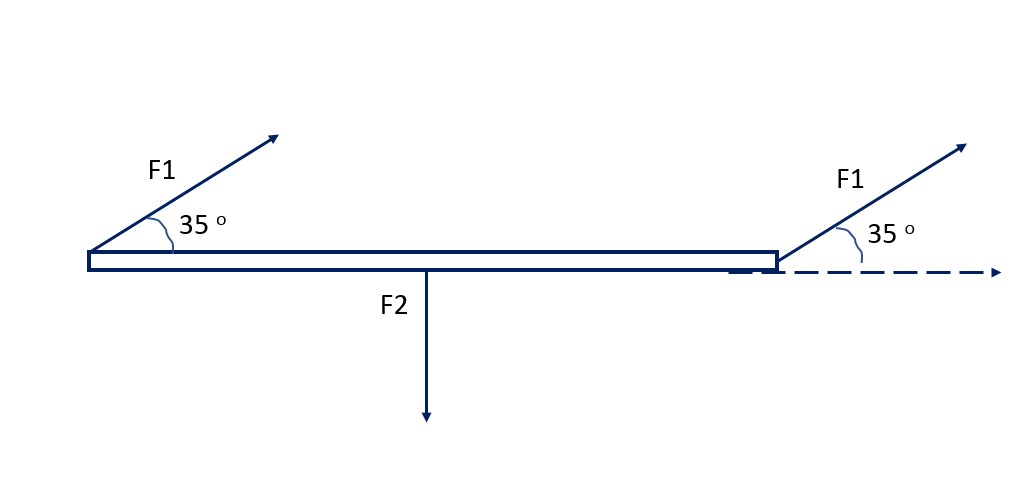
The drawing shows a bar lying on the surface of a horizontal table top. The bar is pivoted at the left end and is free to rotate with negligible friction in the horizontal plane of the table-top about the axis through the end. The bar is 2.0m long and has a mass of 0.60 kg.
The forces are as follows:
F1 = 14.0 N (at the pivot)
F2 = 12.0 N (at the 1.25m mark perpendicular to the bar)
F3 = 14.0 N (at the 2.0m mark)

1 Answer
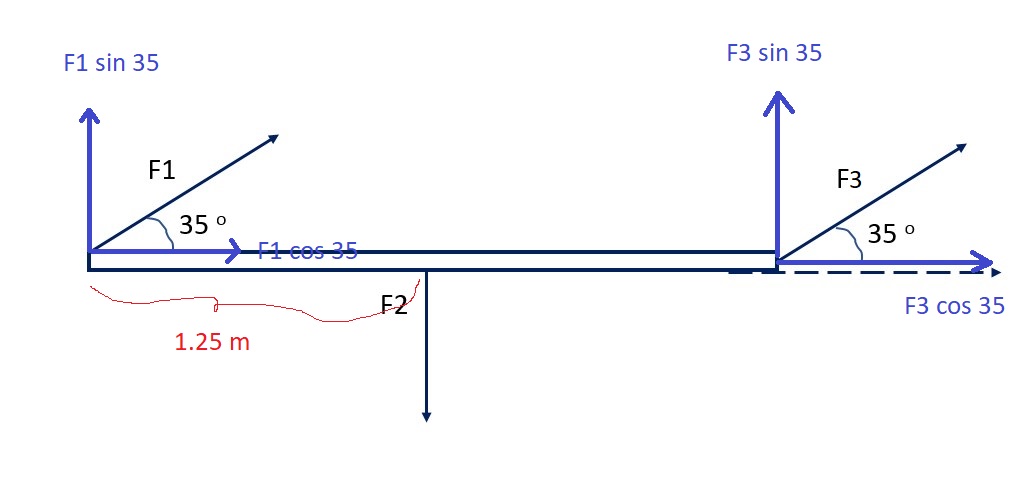
Dividing forces
Clearly,
So,only
Now,considering torque about the pivoted point,and taking the direction of the torque coming out of the plane of the page to be positive, we can write,
Given,
So,net torque acting is
Now,
Given,
So,
Note,here the pivoted point should have enough ability to pull the rod with a force of

