For what values of x is # f(x) = -sqrt(x^3-9x^2 # concave or convex?
1 Answer
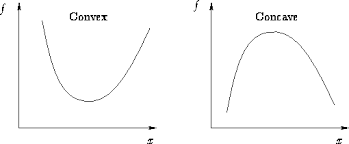
It is concave if the second derivative is less than zero and convex if the second derivative is greater than zero. "How to" is below, numeric solution is left to the student.
Explanation:
An inflection point is a point on a curve at which the sign of the curvature (i.e., the concavity) changes. Inflection points may be stationary points, but are not local maxima or local minima. For example, for the curve
First Derivative Test
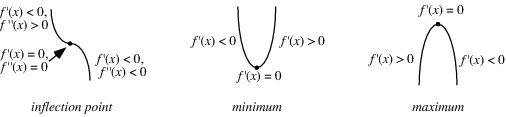
Suppose
1. If
2. If
3. If
Weisstein, Eric W. "First Derivative Test." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/FirstDerivativeTest.html
Second Derivative Test
Suppose
1. If
2. If
The extremum test gives slightly more general conditions under which a function with
If
Define the second derivative test discriminant as
Then
1. If D>0 and
2. If D>0 and
3. If D<0, the point is a saddle point.
4. If D=0, higher order tests must be used.
Weisstein, Eric W. "Second Derivative Test." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/SecondDerivativeTest.html
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 14, 1972.
Thomas, G. B. Jr. and Finney, R. L. "Maxima, Minima, and Saddle Points." §12.8 in Calculus and Analytic Geometry, 8th ed. Reading, MA: Addison-Wesley, pp. 881-891, 1992.

