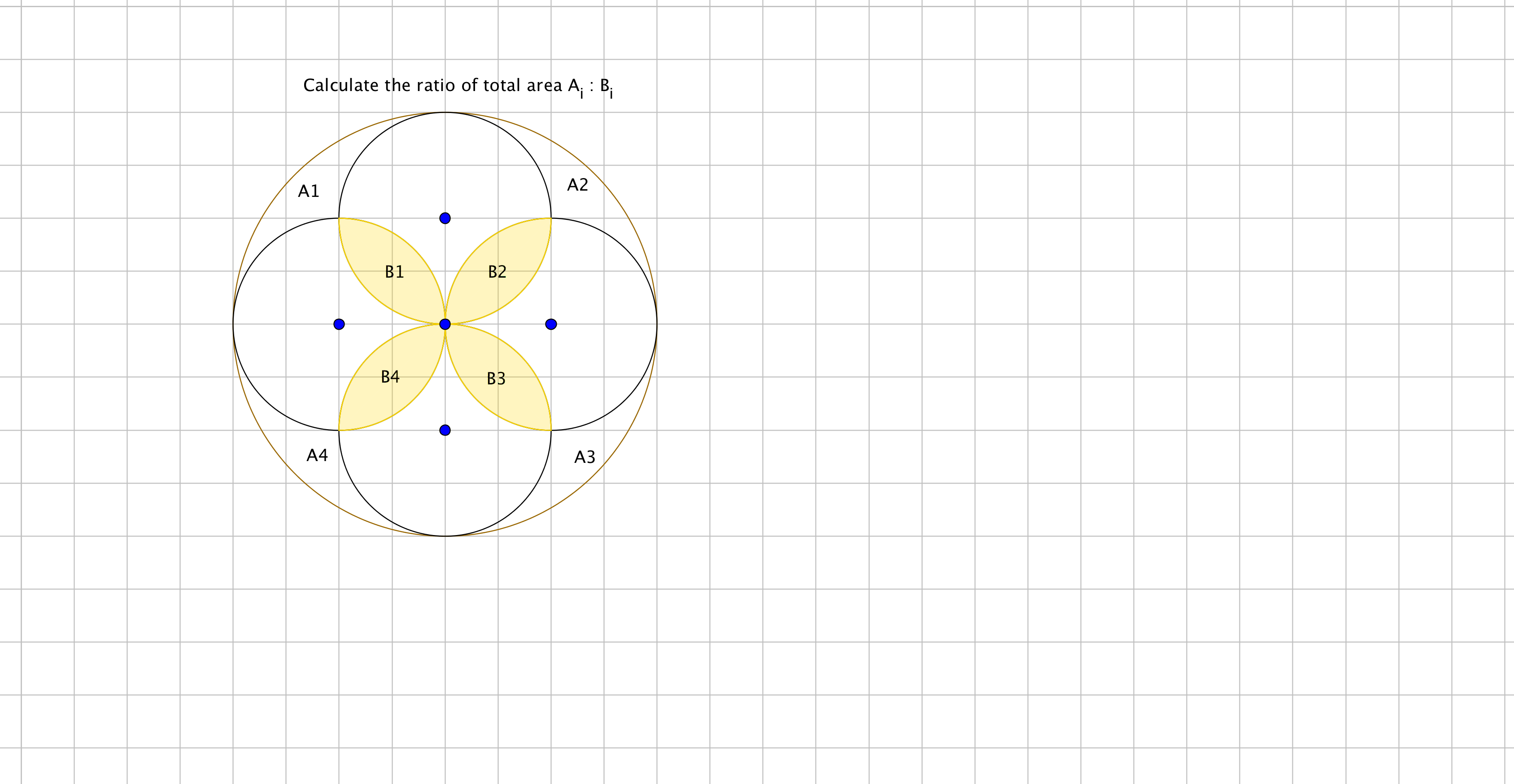
Given that the radius of the circumcircle (large circle) is #r#, evaluate the ratio of the area of A regions to B regions - #(sumA_i)/(sumB_i)#?
1 Answer
Nov 9, 2016
Radius of the large circumcircle
Area of this large circle
The length of each side of the square at the center is equal to the radius of the large circumcircle.
So area of the square
Radius of each of four small circle
So its area
From the figure it is obvious that
Each of the petal of flower at the center is composed of two similar segments of small circle and 8 such segments form the complete flower.
Area of each such segment
So
So the asked ratio

