Question

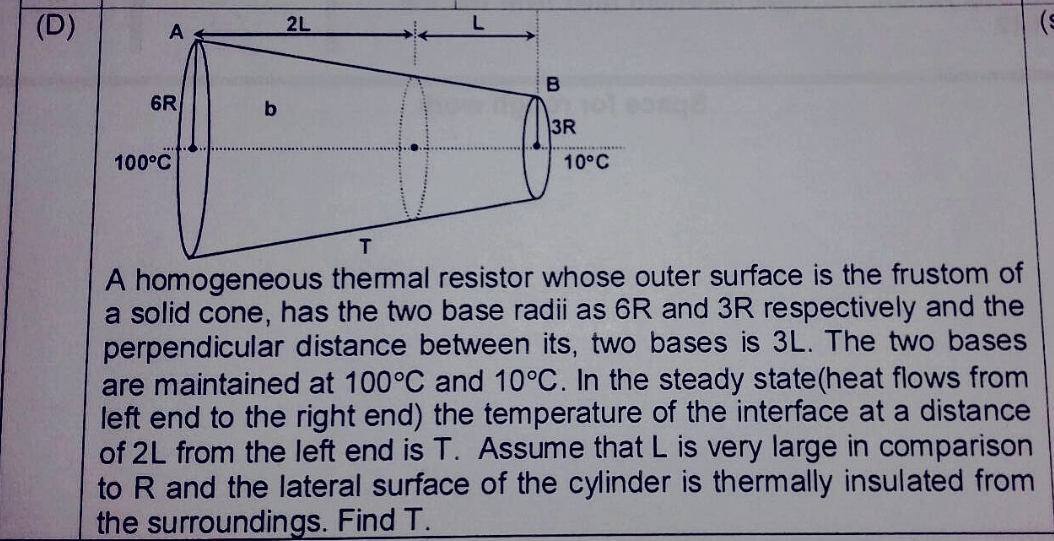
Heat transfer... variable area. ?

Answer 1

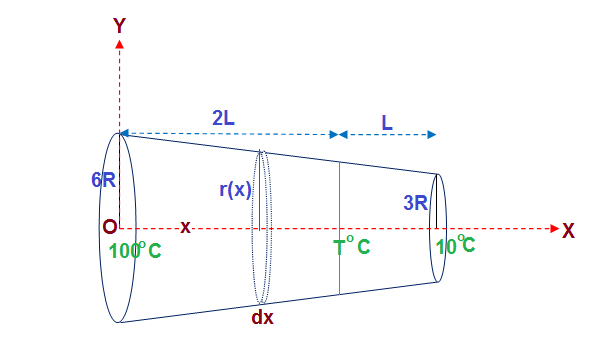
For the sake of computation let us consider that the the center of the left circular surface as origin `(0,0)` and line perpendicular on it through its center point as `x`-axis. We also consider a very thin disk of radius `r(x)` and thickness `dx` at an arbitrary distance `x` from the origin along X- axis.

Here radius of the thermal resistor decreases with respect to its length at the rate of `=(3R-6R)/(3L)=-R/L`.
Expressing radius of circular cross section as function of `x` as

`r(x)=(6 R-R/Lx)`

And area of cross section as function of `x`

`A(x)=pi(6R-R/Lx)^2` .......(1)

Now let `Q` be the rate of heat transfer in one dimension along the axis considered in the steady state .

So the rate of heat transfer through a slice of infinitesimal thickness `dx` at a distance `x` from origin will be-

`Q=-(A(x)kd theta)/(dx)`
where `d theta` represents decrease in temperature across the slice `dx` considered. Here `k` represents the thermal conductivity of the material of the truncated cone.

So

`Q(dx)/(A(x))=-kd theta`

Using (1) we get

`color(magenta)(Q/pi(dx)/(6R-R/Lx)^2=-kd theta)` ......(2)

To find temperature at a particular value of `x` we need to integrate both sides with respect to respective variables. Before that we make following substitution.

Let `z=6R-R/Lx`

`dz=-R/L dx`

With this substitution (2) becomes

`-(QL)/(Rpi)(dz)/z^2=-kd theta`

`=>(QL)/(Rpi)(dz)/z^2=kd theta` .......(3)

The revised required limits for integration are
For `x=0,z=6R`
for `x=2L,z=4R`
and when `x=3L,z=3R`

Integrating (3) for first set of limits we get

`(QL)/(Rpi)int_(6R)^(4R)(dz)/z^2=kint_100^Td theta`
`=>(-QL)/(Rpi)[1/z]_(6R)^(4R)=k[theta]_100^T`
`=>(-QL)/(Rpi)[1/(4R)-1/(6R)]=k(T-100)`
`=>color(blue)((-QL)/(Rpi)[1/(12R)]=k(T-100))` ........(4)

Similarly for second set of limits we have

`(QL)/(Rpi)int_(4R)^(3R)(dz)/z^2=kint_T^10 d theta`
`=>(-QL)/(Rpi)[1/z]_(4R)^(3R)=k[theta]_T ^10`
`=>(-QL)/(Rpi)[1/(3R)-1/(4R)]=k(10-T)`
`=>color(green)((-QL)/(Rpi)[1/(12R)]=k(10-T))` .......(5)

Observing that LHSs of (4) and (5) are equal. So equating RHSs which must also be equal we get

`k(T-100)=k(10-T)`

`T-100=10-T`

`=>color(red)(T=55^@C)`