Question

How do I solve for the points of inflection involving trig functions?

`f(x)=sinx+cosx`, `[0, 2π]`

For the derivative I got:
`f'(x)=cosx-sinx`
`f''(x)=-sinx-cosx`
but after that I get lost. Can you please help me?

Answer 1

The points of inflection are at:
`((3pi)/4,0)` and `((7pi)/4,0)`

Explanation:

You were definitely on the right track. In fact, you had two steps remaining. Points of inflection on a graph are where the concavity of the graph changes. In this case, you're looking for the inflection point of:

`f(x)=sinx+cosx` on the interval of `[0, 2pi]`.

The inflection point comes from where the second derivative is equal to 0. So we need to take the derivative twice, which you did perfectly.

`f'(x)=cosx-sinx`
`f''(x)=-sinx-cosx`

Now, it's a matter of seeing where they're equal to one another. So let's solve for x.

`0=-sinx-cosx`
`cosx=-sinx`

Because of the unit circle, we know the value has to be in the second or fourth quadrants, so x will be between `pi/2` and `pi`, and between `3pi/2` and `2pi`. Considering the values are supposed to be equal, we can easily find the solutions, thanks to the unit circle.

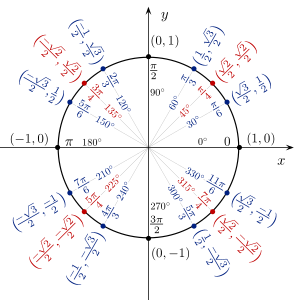
We can see in the image that the functions will be equal at:

`x=(3pi)/4` and `x=(7pi)/4`

So bringing us back to the original question of finding the inflection points, these points are the x values of your inflection points. Now, all you have to do is plug in the values for x into the original function to get your two inflection points. Though considering the second derivative is just the original function multiplied by -1, we can see the points of inflection are at:

`((3pi)/4,0)` and `((7pi)/4,0)`