How do you evaluate #(56)^(7/2)#?

Question

1615 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-10-07T13:27:50

#351232sqrt(14)" "# as an exact value

#1314189.807" "# to 3 decimal places as an approximate value

First of all lets do this using logs:

Let #x=(56)^(7/2)#

#" "log(x)" "=" "log[ (56)^(7/2)]" " =" " 7/2log(56)~~6.1186...#

#=>color(green)(x=log^(-1)(6.1186..)~~1314189.807)# to 3 decimal places

#color(blue)("This is not a precise solution but will do as a check.")#
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Consider again #x=(56)^(7/2)#

This is the same as: #sqrt(56^7)" "=" "sqrt(56^2xx56^2xx56^2xx56)#

#=56^3sqrt(56)#

Splitting 56 into a product of primes we observe:
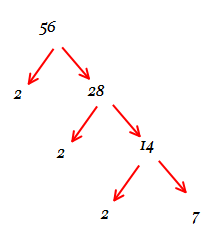
Tony B

Giving:

#56^3sqrt(2^2xx14)#

#2xx56^3sqrt(14)color(green)(~~1314189.807)# to 3 decimal places

#color(blue)("So the exact value is: "351232sqrt(14))#