How do you evaluate sine, cosine, tangent of #-pi/6# without using a calculator?
2 Answers
Explanation:
Recall that:
and,
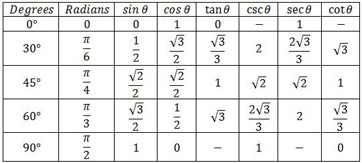
Hence,
Explanation:
Draw an equilateral triangle with unit lengths. Each angle is
Now, bisect one of the angles. This gives two right triangles.
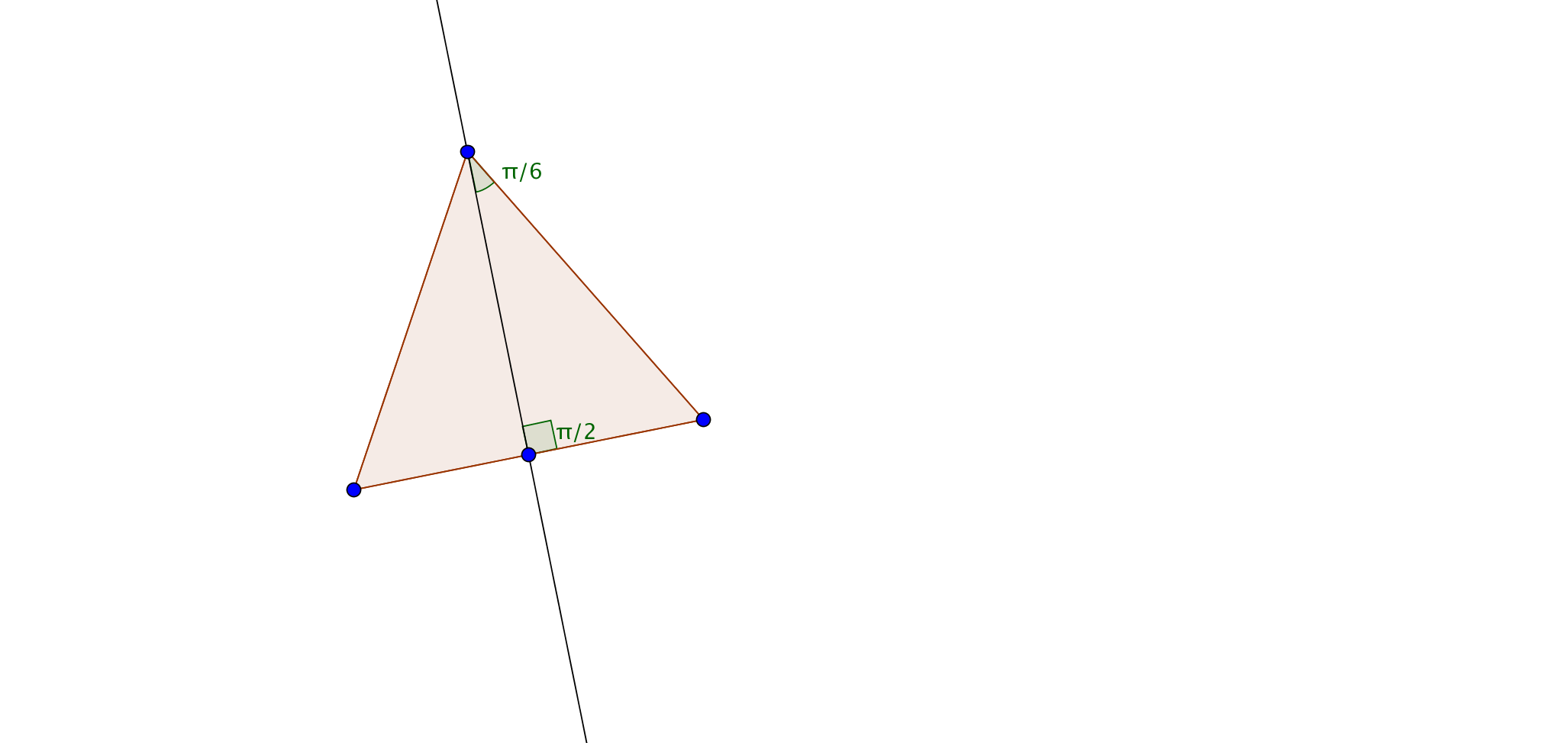
Look at the right triangle to the right. The hypotenuse is
Then,
Recall the following identities (or use a unit circle):
Thus,


