Question

How do you factor the trinomial `n^2 - 10n - 25`?

Answer 1

For the given question factorization would give:
`(n+2.071068)( n-12.071068)`

If the equation had been `n^2+10n+25 -> (n+5)^2`
`color(blue)("I have asked some one else to have a look at this!")`

Explanation:

`color(blue)("Step 1")`
There is no coefficient in front of `n^2` so our starting point is:

`(n+?)(n+?)`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("Step 2")`
Consider the constant of `-25`

The constant is the product of two numbers and as the 25 is negative that must mean that one of them is positive and the other negative.

Integer factors of 25 are: {1,25} ; {5,5}

The thing is, `(-5)xx5=-25` as required but `(-5)+5!=-10`

`color(brown)("So I can not help but asks: Is the question correct?")`

Suppose the question was: `n^2+10n+25` then the factorization would be: `(n+5)^2`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("Step 3")`
Investigate key points of the graph

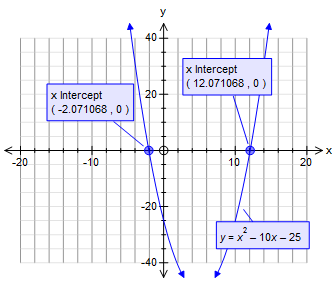
Using the standard form of `y=ax^2+bx+c"`
and `x=(-b+-sqrt(b^2-4ac))/(2a)`

Will give the values shown on the graph

Consequently the factors for the given equation will be:

`(n+2.071068)( n-12.071068)`

Answer 2

Since D = b^2 - 4ac = 100 + 100 = 200 is not a perfect square, therefor, the trinomial can't be factored.