Question

How do you find the domain and range of `y = |x - 6|`?

Answer 1

Domain`color(blue)( = (-oo, +oo)`

Range`color(blue)( = [0, +oo)`

Explanation:

We are give the absolute Value Function `y = f(x) = |x-6|`

Investigate the graph below:

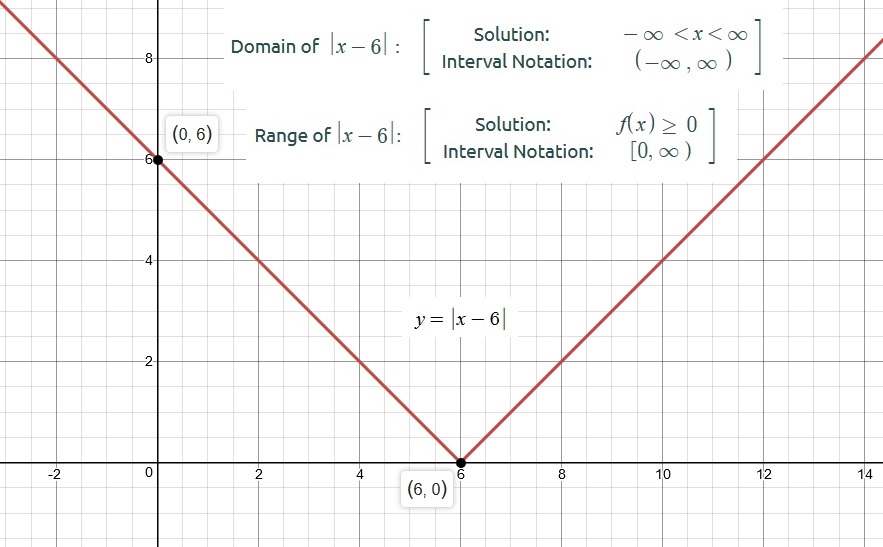
We observe that ALL Real Values are possible for the function's Domain `x`

Hence, Domain `= -oo < x < +oo`

We can also write the Domain as `(-oo, +oo)`

For `y`, the function's Range is all values `f(x)>= 0`

Hence, Range `= fx) >=0`

We can also write the Range as `[0, oo)`

I Hope you find this solution useful.