Since #y=f(x)=(3-2x)/(x+2)=(-2x+3)/(x+2)# is a rational function where the degree of the numerator and denominator are the same (they're both linear with degree 1), we can describe the end behavior by looking at the ratio of the coefficients, #-2# and #1#, of the highest powers of #x# in the numerator and denominator, which is #(-2)/1=-2#.
This implies that #y->-2# as #x-> pm infty# or, in limit notation, #lim_{x->pm infty}f(x)=2#. This means that the horizontal line #y=2# is a horizontal asymptote of the graph of #f# as #x# gets farther and farther away from zero.
A bit more of an "official" way to calculate this limit is to show the following steps (with the initial step involving a "trick" that allows us to bring the limit sign into the various pieces of the function):
#lim_{x->pm infty}f(x)=lim_{x->pm infty} (-2x+3)/(x+2) * (1/x)/(1/x)#
#=lim_{x->pm infty}(-2+3/x)/(1+2/x)=(lim_{x->pm infty}(-2)+3*lim_{x->pm infty} 1/x)/(lim_{x->pm infty}1+2*lim_{x->pm infty} 1/x)#
(in reality, a merged a bunch of steps into one step for this last equality).
Since #lim_{x->pm infty}c=c# and #lim_{x->pm infty} 1/x=0# (stated without proof), it follows that
#=(lim_{x->pm infty}(-2)+3*lim_{x->pm infty} 1/x)/(lim_{x->pm infty}1+2*lim_{x->pm infty} 1/x)=(-2+3*0)/(1+2*0)=-2#
Here's a picture of the function and its horizontal asymptote:
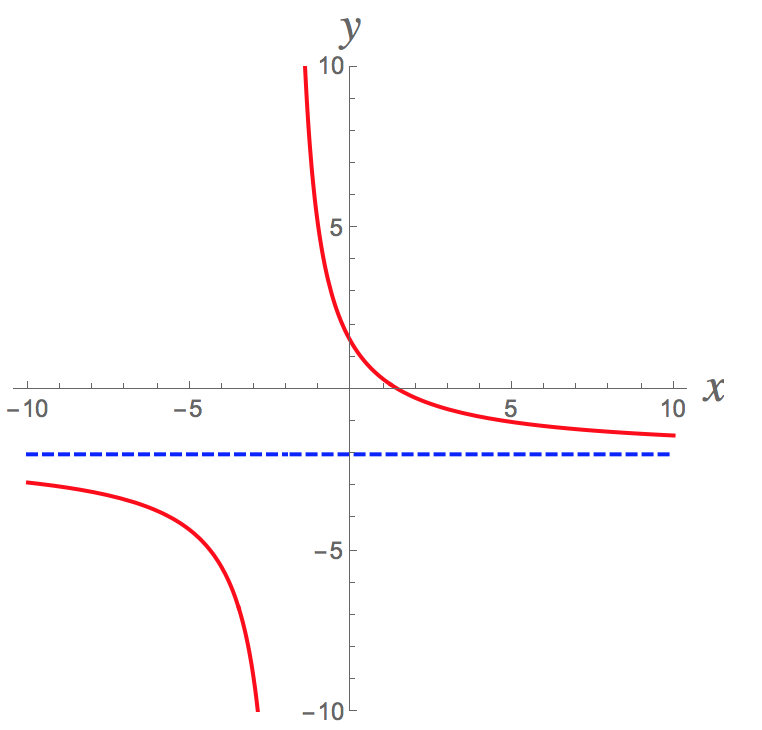
An interesting feature of this function is that its graph appears to have symmetry with respect to the diagonal line #y=x# (not shown). In other words, the function and its inverse appear to have the same graph. Appearances can be deceiving, however, so you should see if you can algebraically demonstrate that #f^{-1}(x)=f(x)# for this function (it is true, by the way).


