Question

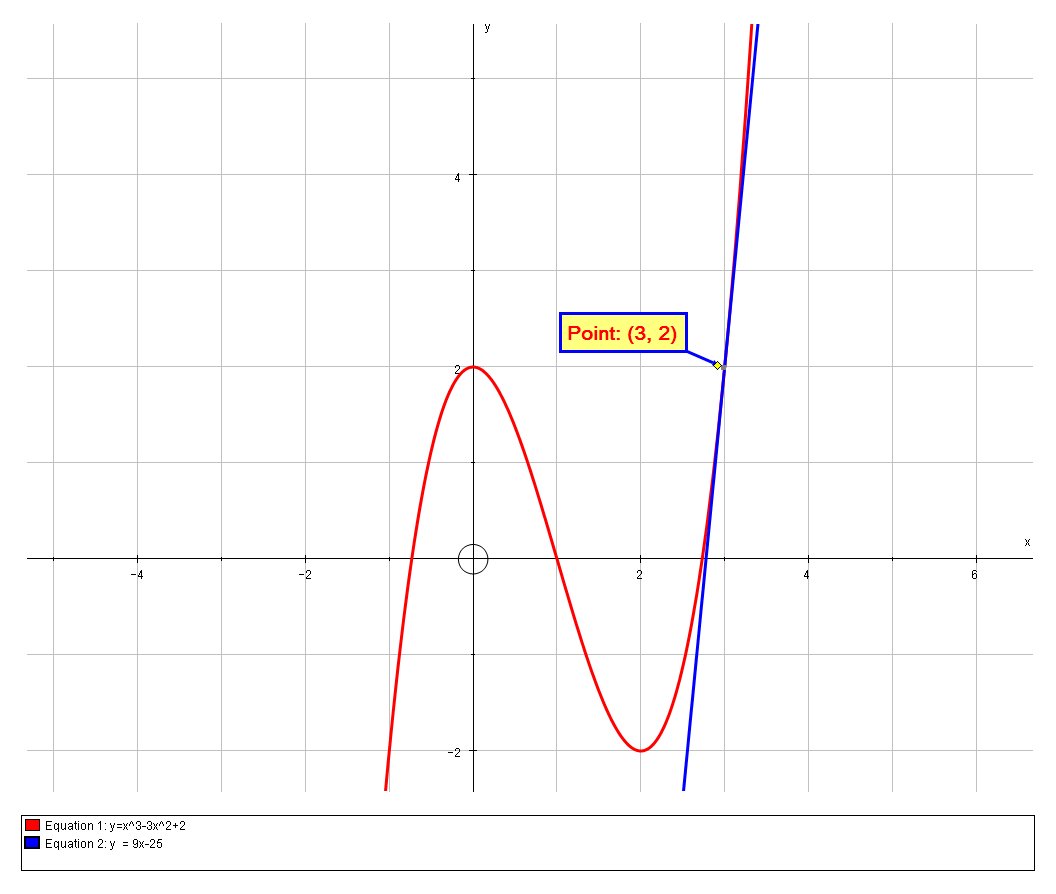
How do you find the equation of a line tangent to the function `y=x^3-3x^2+2` at (3,2)?

Answer 1

`y = 9x-25`

Explanation:

We have a curve given by the equation:

`y=x^3-3x^2+2`

The gradient of the tangent to a curve at any particular point is given by the derivative of the curve at that point. So if we differentiate the equation we have:

`dy/dx = 3x^2-6x`

And so the gradient of the tangent at `(3.2)` is given by:

`m = [dy/dx]_(x=3)`
`\ \ = 27-18`
`\ \ = 9`

So, using the point/slope form `y-y_1=m(x-x_1)` the tangent equations is;

`y - 2 = 9(x-3)`
`:. y - 2 =9x-27`
`:. y = 9x-25`

We can verify this solution graphically: