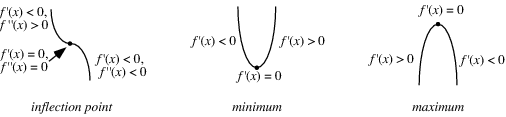How do you find the local extrema for #F(x)= sin (x + (π/2) )#?

1 Answer
SCooke
Sep 9, 2017

Local extrema are where the first derivative of the expression is equal to zero.

Explanation:

The first derivative of an expression is an inflection point of the expression wherever it is equal to zero. It is a local maxima or minima (extrema) depending on whether the second derivative is positive or negative. IF the second derivative changes sign, then the point is an inflection point and not an extrema.

This can also be determined from the change in sign of the first derivative around the point.
enter image source here

Related questions
See all questions in Identifying Turning Points (Local Extrema) for a Function
Impact of this question
1604 views around the world
You can reuse this answer
Creative Commons License