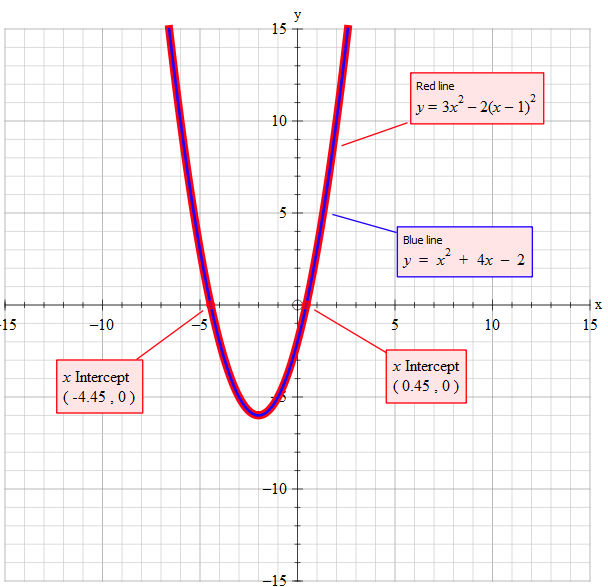
How do you find the roots, real and imaginary, of #y= 3x^2-2(x- 1 )^2 # using the quadratic formula?

Question

1367 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-04-12T10:46:38

There are no imaginary roots as the graph has x-intercepts.

#" "x~~-4.45 and x~~0.45# to 2 decimal places

First we need to change the given equation so that it is in the form #y=ax^2+bx+c#

Square the brackets

#y=3x^2-2(x^2-2x+1)#

#y=3x^2-2x^2+4x-2#

#y=x^2+4x-2#

To determine the roots set #y=0#

#0=x^2+4x-2#

So we have: #a=1"; "b=4"; "c=-2#

#x=(-b+-sqrt(b^2-4ac))/(2a)" "->x=(-4+-sqrt((-4)^2-4(1)(-2)))/(2(1))#

#" "x=-2+-sqrt(16+8)/2#

#" "x=-2+-sqrt(24)/2#

But # 24=2xx3xx4# so #sqrt(24)=2sqrt(6)#

#" "x=-2+-(2sqrt(6))/2#

#" "x=-2+-sqrt(6)#

#" "x~~-4.45 and x~~0.45#
Tony B