Question

How do you find the roots, real and imaginary, of `y=-5(x-3)^2-45` using the quadratic formula?

Answer 1

`x=-3/2+-9/2i`

Explanation:

To make this more strait forward lets convert the equation back to the form of `y=ax^2+bx+c`

Square the brackets.

`y=-5(x^2-3x+9)-45`

Multiply out the -5

`y=-5x^2+15x-45-45`

`y=-5x^2+15x-90`

Thus the quadratic formula states that

`x=(-b+-sqrt(b^2-4ac))/(2a)`

where `a=-5"; "b=+15"; "c=-90`

`x=(-15+-sqrt((-15)^2-4(-5)(-90)))/(2(-5))`

`x=-3/2+-(sqrt(-2025))/(-10)`

If you are ever not sure about factors do a quick sketch of a prime factor tree on the side of the page.

`x=-3/2+-(sqrt(5^2xx9^2xx(-1)))/(-10)`

`x=-3/2+-( cancel(5)^1xx9xxi)/(cancel(10)^2)`

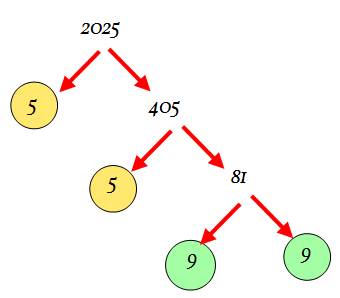
As there is a `+-` sign in front of `(9xxi)/2` you can ignore any negative within it.

`x=-3/2+-9/2i`