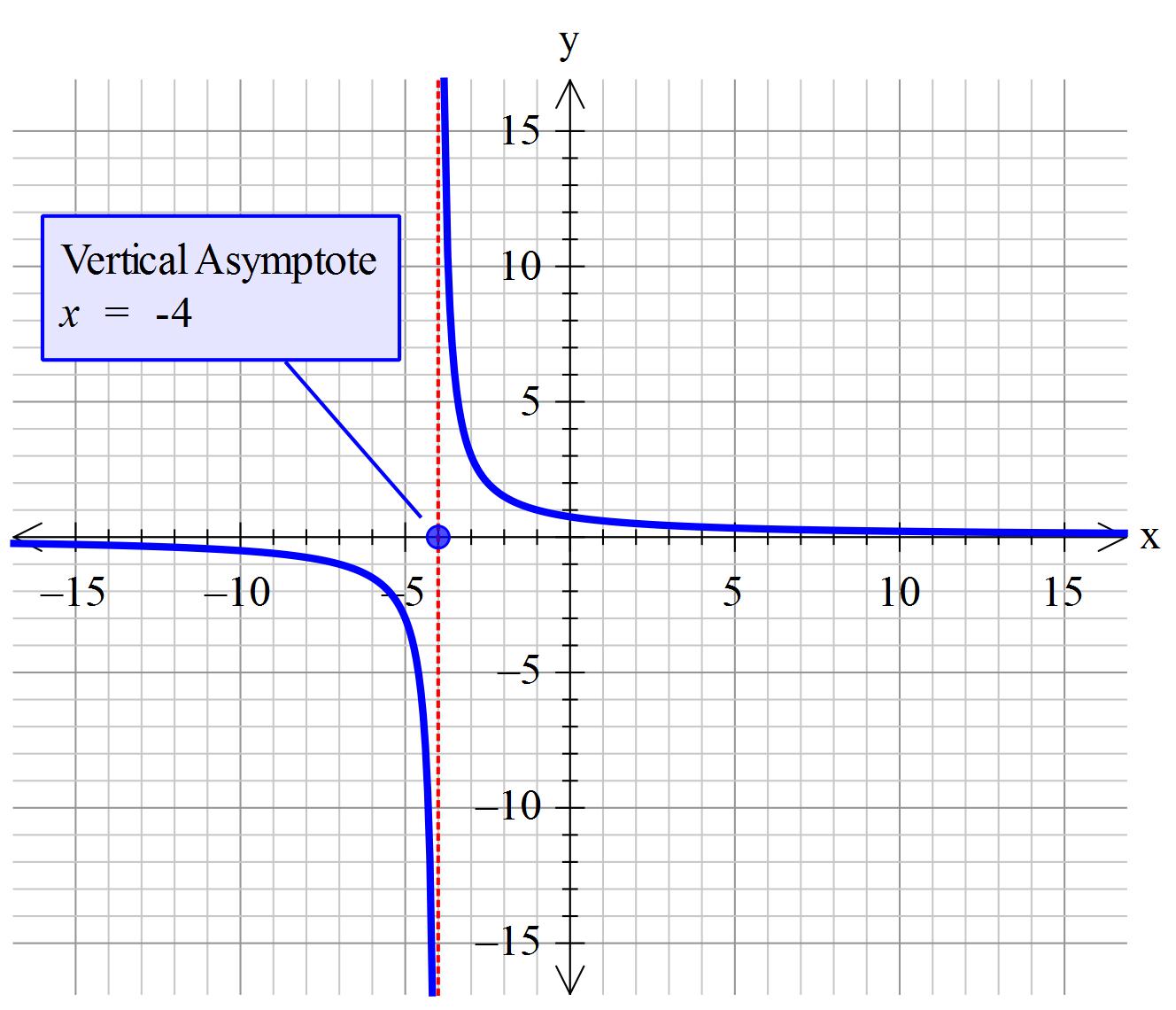
How do you find the vertical, horizontal or slant asymptotes for #y = 3/(x + 4)#?
1 Answer
May 31, 2016
Vertical asymptote is
Horizontal asymptote is
Explanation:
The equation is undefined at
This
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
As the magnitude of
Thus