How do you find the volume bounded by #y=ln(x)# and the lines y=0, x=2 revolved about the y-axis?
2 Answers
For the solution by cylindrical shells, see below.
Explanation:
Here is a picture of the region and a representative slice taken parallel to the axis of rotation.
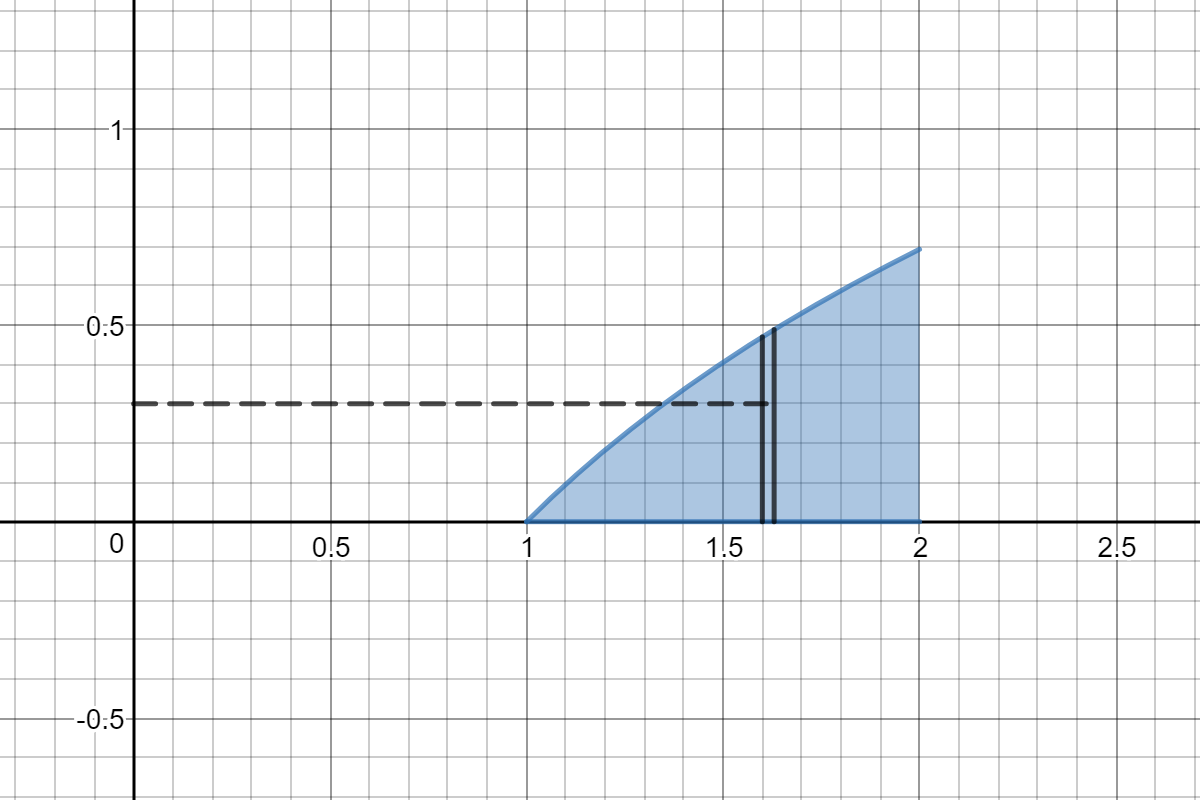
The slice is taken at some value of
Revolving about the
The volume of this representative shell is
The radius is shown as a dashed black line in the picture and has length
The height of the shell will be the great
As already mentioned, the thickness is
The representative volume is
Use integration by parts to get
# = 2pi(2ln2-3/4)#
(Rewrite the answer to taste.)
For the solution by washers see below.
Explanation:
Here is a picture of the region and a representative slice taken perpendicular to the axis of rotation.

The slice is taken at some value of
The curve
Revolving about the
The volume of this representative washer is
Where
In this case
and
The representative volume is
so
And the volume is
Use integration to get
# = pi[(4ln2-1/2e^(2ln2))-(-1/2e^0)] = pi(4ln2-3/2)#
(Rewrite the answer to taste.)

