Question

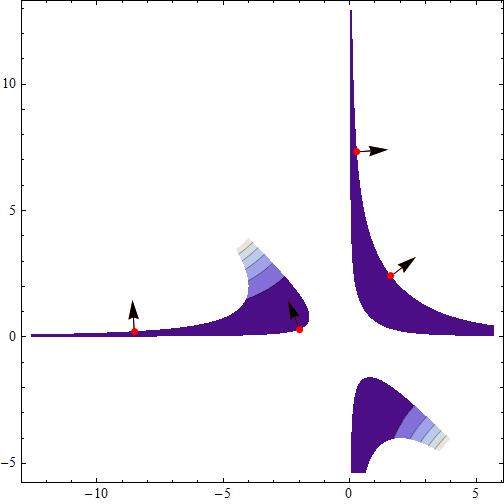
How do you minimize and maximize `f(x,y)=(xy)^2-x+y` constrained to `1<yx^2+xy^2<16`?

Answer 1

See below.

Explanation:

There are four characteristic points characterizing local maxima/minima at

# ((f(x,y), x, y,"type"), (2.63539,-1.9786,0.301326,"maximum"), (11.4563,0.286781,7.32736,"minimum"), (12.4683,-8.52656,0.226069,"maximum"), (16.382,1.63582,2.41474,"minimum")) #

This result can be obtained using the Lagrange Multipliers technique. The attached plot shows the feasible region with the points located at the boundaries.