How do you prove that a shape is a kite?

1 Answer
sankarankalyanam
Mar 2, 2018

Kite properties :
Two pairs of sides are of equal length.
One pair of diagonally opposite angles is equal.
Only one diagonal is bisected by the other.
The diagonals cross at 90°

Explanation:

Properties of a kite :

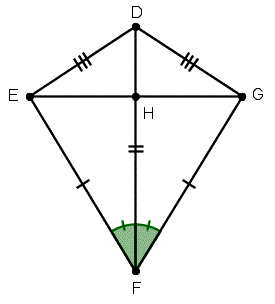
enter image source here

  1. Two pairs of adjacent sides are equal.
    #EF = GF, ED = GD#

  2. Hence diagonal FD is the angular bisector of angles #hatF, hatD#

  3. Diagonals intersect at right angles.
    #FD# perpendicular #EG#

  4. Shorter diagonal is bisected by the longer diagonal.
    #EH = HG#

  5. Only one pair of opposite angles is equal.
    #hatE = hatG#

All the above 5 conditions are to be satisfied for a quadrilateral to be called a KITE

Related questions
Impact of this question
20732 views around the world
You can reuse this answer
Creative Commons License