Question

How do you simplify `9(2y-4)-2(7y-12)`?

Answer 1

`4y -12`

Explanation:

`9(2y-4) - 2(7y-12)`

To simplify this, use the distributive property:

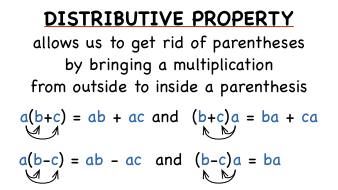
Following this image, we know that:
`color(blue)(9(2y-4) = (9 * 2y) + (9 * -4) = 18y - 36)`
and
`color(blue)(-2(7y-12) = (-2 * 7y) + (-2 * -12) = -14y + 24)`

Therefore, the expression becomes:
`18y - 36 - 14y + 24`

Combine the like terms:
`4y -12`

Hope this helps!

Answer 2

`4(y-3)`

Explanation:

Let's distribute the `9` and `-2` to their respective terms. We get

`18y-36-14y+24`

Next, we can combine our `y` terms to get `4y` and our constants to get `-12`. Thus, we have

`4y-12`

We can recognize that both terms have a `4` in common, so we can factor that out to get

`4(y-3)`

Hope this helps!