#4(b+6) = 2(b+5) + 2#
To solve for the variable #b#, we need to make it by itself. First, use the distributive property (shown below) to simplify #4(b+6)# and #2(b+5)#:
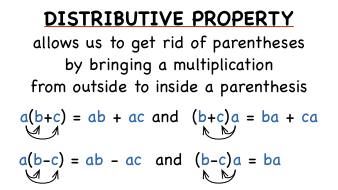
Following this image, we know that:
#color(blue)(4(b+6) = (4 * b) + (4 * 6) = 4b + 24)#
and
#color(blue)(2(b+5) = (2 * b) + (2 * 5) = 2b + 10)#
Put them back into the equation:
#4b + 24 = 2b + 10 + 2#
Combine #10 + 2#:
#4b + 24 = 2b + 12#
Subtract #color(blue)(2b)# from both sides:
#4b + 24 quadcolor(blue)(-quad2b) = 2b + 12 quadcolor(blue)(-quad2b)#
#2b + 24 = 12#
Now subtract #color(blue)24# from both sides:
#2b + 24 quadcolor(blue)(-quad24) = 12 quadcolor(blue)(-quad24)#
#2b = -12#
Divide both sides by #color(blue)2#:
#(2b)/color(blue)2 = -12/color(blue)2#
Therefore,
#b = -6#
Hope this helps!


