Question

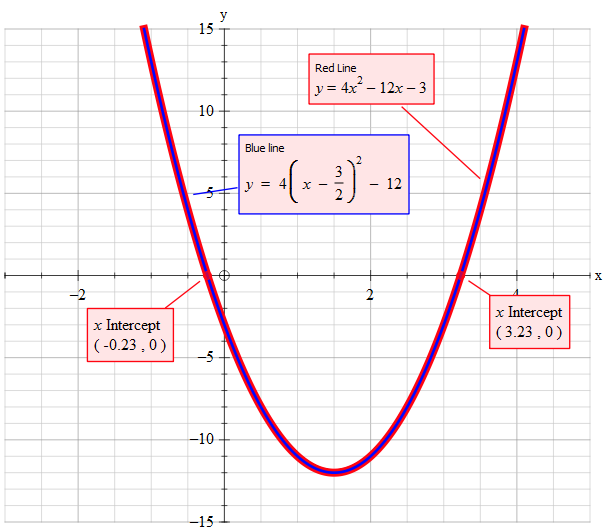
How do you solve `4x^2 - 12x - 3 = 0` by completing the square?

Answer 1

`x=3/2+-sqrt(3)`

`x~~-0.23 and x~~+3.23`

Explanation:

Given:`" "0=4x^2-12x-3`

For a complete explanation of the method see:
https://socratic.org/s/aFvmbGAs

It uses different numbers.

Set `y=0=4x^2-12x-3`

`y=0=4(x-12/(4xx2))^2+k-3`

`y=0=4(x-3/2)^2+k-3`
.........................................................................

Set `" "4(-3/2)^2+k=0`

`4xx9/4+k=0" "=>" "k =-9`
.......................................................................

`y=0=4(x-3/2)^2-12`

`+12/4=(x-3/2)^2`

`x-3/2=+-sqrt(3)`

Exact answer is:
`x=3/2+-sqrt(3)`

Approximate answer is:
`x=-0.232050.. and x=+3.232-50....`