Question

How do you solve `-5=-2 (x+1)-2(2- x)`?

Answer 1

No Solution or `cancel(0)`.

Explanation:

`-5 = -2(x+1) - 2(2-x)`

This is how you distribute something:

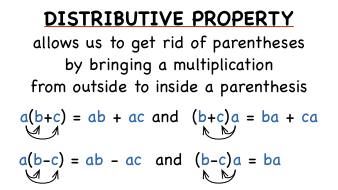
Following this image, we know that:
`-2(x+1) = -2 * x - 2 * 1 = -2x - 2`

`-2(2-x) = -2 * 2 - 2 * -x = -4 + 2x`

Now let's put these expressions back into the equation:
`-5 = -2x-2 - 4 + 2x`

Let's color code the like terms on the right side of the equation:
`-5 = color(magenta)(-quad2x) quadcolor(blue)(-quad2quad-quad4) quadcolor(magenta)(+quad2x)`

Combine like terms:
`-5 = -6`

However, now we don't have a variable to solve for! Now we look at whether this equation is true. Is it true that `-5 = -6`? NO!

That means the answer is No Solution or `cancel(0)`.

Hope this helps!