How do you solve #lnx=-3#?
1 Answer
Jan 7, 2017
Explanation:
Let's use the diagram below: 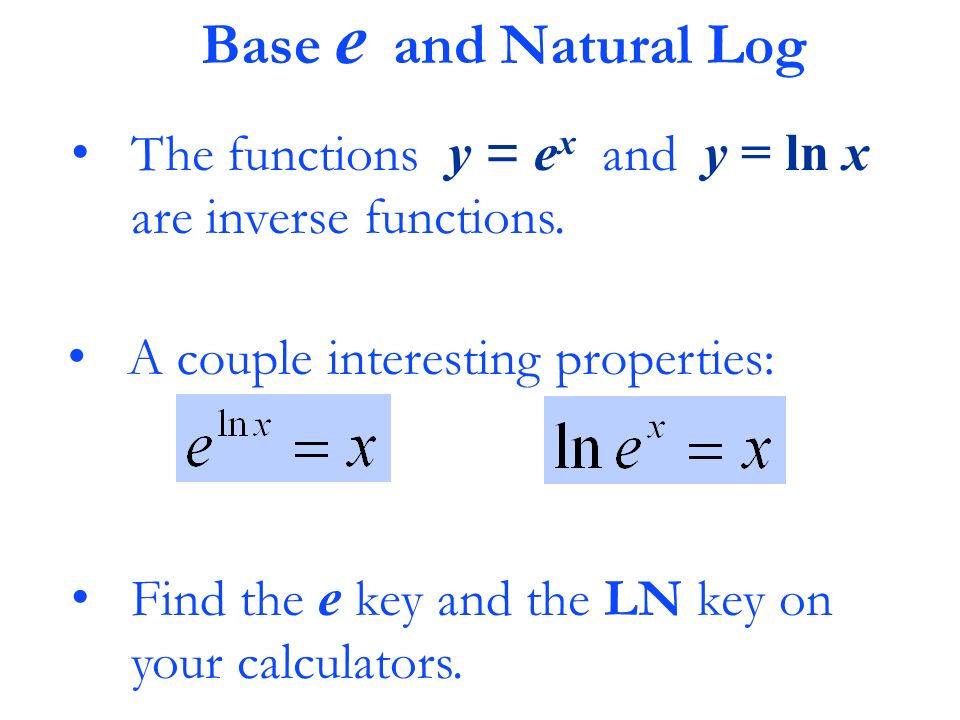
This photo tells us that the natural log (ln) and the exponential function (
In our case if we raise e to the ln x, we are just left with x on the left side since
Now, we have to do the same thing on the right side and raise e to the -3 power like this:
When you do that calculation, you obtain an approximate value of .050.

