How do you solve the equation #3sqrt3tanu=3#?
1 Answer
Apr 7, 2017
x is an integer
Explanation:
Some concepts needed when finding u:
Math:
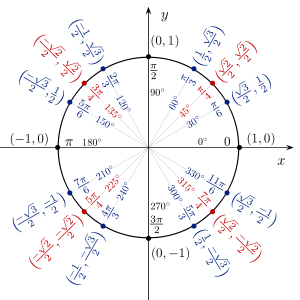
This is because the ratio which fits one over square root of three is one half over two divided by square root of three since the twos cancel out. Negatives also cancel, which is why there are 2 answers.
In reality, there would be an infinite amount of solutions with equations since angles can keep going around. So since a full rotation is

